A $2 \mathrm{~kg}$ steel rod of length $0.6 \mathrm{~m}$ is clamped on a table vertically at its lower end and is free to rotate in vertical plane. The upper end is pushed so that the rod falls under gravity, Ignoring the friction due to clamping at its lower end, the speed of the free end of rod when it passes through its lowest position is ...........ms ${ }^{-1}$. (Take $g=10 \mathrm{~ms}^{-2}$ )
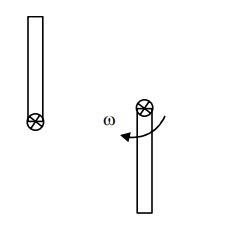
by energy conservation $\mathrm{mg} \ell=\frac{1}{2} \mathrm{I} \omega^{2}=\frac{1}{2} \frac{\mathrm{m} \ell^{2} \omega^{2}}{3}$
$\Rightarrow \omega=\sqrt{\frac{6 g}{\ell}}$
Speed $\mathrm{v}=\omega \mathrm{r}=\omega \ell=\sqrt{6 \mathrm{~g} \ell}$
$v=\sqrt{6 \times 10 \times .6}=6 \mathrm{~m} / \mathrm{s}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
