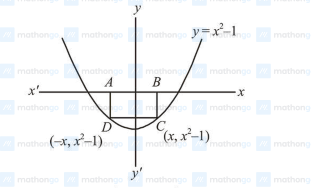
The area (in sq. units) of the largest rectangle $A B C D$ whose vertices $A$ and $B$ lie on the $x$-axis and vertices $C$ and $D$ lie on the parabola, $y=x^{2}-1$ below the $x$-axis, is :
Correct Option:
Area of rectangle $A B C D$
$A=2 x \cdot\left(x^{2}-1\right)=2 x^{3}-2 x$
$\therefore \quad \frac{d A}{d x}=6 x^{2}-2$
For maximum area $\frac{d A}{d x}=0 \Rightarrow x=\pm \frac{1}{\sqrt{3}}$
$\frac{d^{2} A}{d x^{2}}=(12 x) \Rightarrow\left(\frac{d^{2} A}{d x^{2}}\right)_{x=\frac{-1}{\sqrt{3}}}=\frac{-12}{\sqrt{3}}<0$
$\therefore$ Maximum area $=\left|\frac{2}{3 \sqrt{3}}-\frac{2}{\sqrt{3}}\right|=\frac{4}{3 \sqrt{3}}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.