Question:
The area (in sq. units) of the region
$A=\{(x, y):(x-1)[x] \leq y \leq 2 \sqrt{x}, 0 \leq x \leq 2\}$
where $[t]$ denotes the greatest integer function, is :
Correct Option: 1
Solution:
$(x-1)[x] \leq y \leq 2 \sqrt{x}, \quad 0 \leq x \leq 2$
Draw $y=2 \sqrt{x} \Rightarrow y^{2}=4 x \quad x \geq 0$
$y=(x-1)[x]=\left\{\begin{array}{cc}0 & , 0 \leq x<1 \\ x-1, & 1 \leq x<2 \\ 2, & x=2\end{array}\right.$
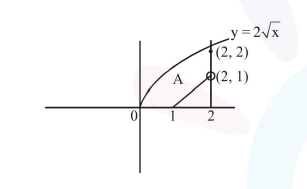
$\mathrm{A}=\int_{0}^{2} 2 \sqrt{\mathrm{x}} \mathrm{dx}-\frac{1}{2} 1 \cdot 1$
$A=2 \cdot\left[\frac{x^{3 / 2}}{(3 / 2)}\right]_{0}^{2}-\frac{1}{2}=\frac{8 \sqrt{2}}{3}-\frac{1}{2}$
