The area of the region

Given $A=\{(x, y): 0 \leq y \leq x|x|+1$ and $-1 \leq x \leq 1\}$

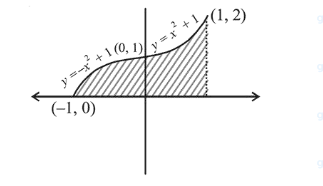
$\therefore$ Area of shaded region

$=\int_{-1}^{0}\left(-x^{2}+1\right) d x+\int_{0}^{1}\left(x^{2}+1\right) d x$

$=\left(-\frac{x^{3}}{3}+x\right)_{-1}^{0}+\left(\frac{x^{3}}{3}+x\right)_{0}^{1}$

$=0-\left(\frac{1}{3}-1\right)+\left(\frac{1}{3}+1\right)-(0+0)$

$=\frac{2}{3}+\frac{4}{3}=\frac{6}{3}=2 \quad$ square units