The areas of two similar triangles are 100 cm2 and 64 cm2 respectively.

Let the two triangles be ABC and PQR with medians AM and PN, respectively.

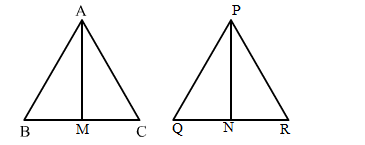
Therefore, the ratio of areas of two similar triangles will be equal to the ratio of squares of their corresponding medians.

$\therefore \frac{\operatorname{ar}(\Delta A B C)}{\operatorname{ar}(\Delta P Q R)}=\frac{A M^{2}}{P N^{2}}$

$\Rightarrow \frac{64}{100}=\frac{5.6^{2}}{P N^{2}}$

$\Rightarrow P N^{2}=\frac{64}{100} \times 5.6^{2}$

$\Rightarrow P N^{2}=\sqrt{\frac{100}{64} \times 5.6 \times 5.6}$

$=7 \mathrm{~cm}$

Hence, the median of the larger triangle is 7 cm.