The base $B C$ of $\triangle A B C$ is divided at $D$ such that $B D=\frac{1}{2} D C$. Prove that $\operatorname{ar}(\Delta A B D)=\frac{1}{3} \times \operatorname{ar}(\Delta A B C)$.
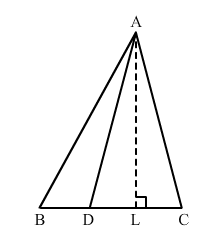
Given: $\mathrm{D}$ is a point on $\mathrm{BC}$ of $\triangle A B C$, such that $\mathrm{BD}=\frac{1}{2} D C$
To prove: $\operatorname{ar}(\Delta A B D)=\frac{1}{3} \operatorname{ar}(\Delta A B C)$
Construction: Draw AL ⊥ BC.
Proof:
In ∆ABC, we have:
BC = BD + DC
⇒ BD + 2 BD = 3 × BD
Now, we have:
$\operatorname{ar}(\Delta A B D)=\frac{1}{2} \times B D \times A L$
$\operatorname{ar}(\Delta A B C)=\frac{1}{2} \times B C \times A L$
$\Rightarrow \operatorname{ar}(\Delta A B C)=\frac{1}{2} \times 3 B D \times A L=3 \times\left(\frac{1}{2} \times B D \times A L\right)$
$\Rightarrow \operatorname{ar}(\Delta A B C)=3 \times \operatorname{ar}(\Delta A B D)$
$\therefore \operatorname{ar}(\Delta A B D)=\frac{1}{3} \operatorname{ar}(\Delta A B C)$
