Question:
The diagonals of a quadrilateral ABCD are equal. Prove that the quadrilateral formed by joining the midpoints of its sides is a rhombus.
Solution:
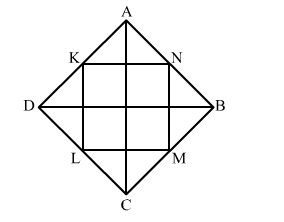
Given: In quadrilateral ABCD, BD = AC and K, L, M and N are the mid-points of AD, CD, BC and AB, respectively.
To prove: KLMN is a rhombus.
Proof:
In ∆ADC,
Since, K and L are the mid-points of sides AD and CD, respectively.
So, $K L \| A C$ and $K L=\frac{1}{2} A C$ ...(1)
Similarly, in ∆ABC,
Since, M and N are the mid-points of sides BC and AB, respectively.
So, $N M \| A C$ and $N M=\frac{1}{2} A C$ ...(2)
From (1) and (2), we get
KL = NM and KL || NM
But this a pair of opposite sides of the quadrilateral KLMN.
So, KLMN is a parallelogram.
Now, in ∆ABD,
Since, K and N are the mid-points of sides AD and AB, respectively.
But this a pair of opposite sides of the quadrilateral KLMN.
So, KLMN is a parallelogram.
Now, in ∆ABD,
Since, K and N are the mid-points of sides AD and AB, respectively.
So, $K N \| B D$ and $K N=\frac{1}{2} B D$ ...(3)
But BD = AC (Given)
$\Rightarrow \frac{1}{2} B D=\frac{1}{2} A C$
$\Rightarrow K N=N M \quad[$ From $(2)$ and $(3)]$
But these are a pair of adjacent sides of the parallelogram KLMN.
Hence, KLMN is a rhombus.
Hence, KLMN is a rhombus.
