Question:
The domain of the definition of the function $f(x)=\frac{1}{4-x^{2}}+\log _{10}\left(x^{3}-x\right)$ is:
Correct Option: , 3
Solution:
To determine domain, denominator $\neq 0$ and $x^{3}-x>0$
i.e., $4-x^{2} \neq 0 x \neq \pm 2$ .......(1)
and $x(x-1)(x+1)>0$
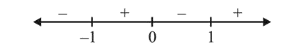
$x \in(-1,0) \cup(1, \infty)$ .......(2)
Hence domain is intersection of $(1) \&(2)$.
i.e., $x \in(-1,0) \cup(1,2) \cup(2, \infty)$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.