The following results show the number of workers and the wages paid to them in two factories $F_{1}$ and $F_{2}$
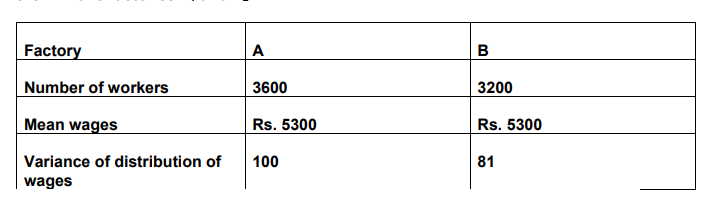
Which factory has more variation in wages?
Mean wages of both the factories are the same, i.e., Rs. 5300.
To compare variation, we need to find out the coefficient of variation (CV).
We know, CV $\frac{\mathrm{SD}}{\text { Mean }} \times 100$ where SD is the standard deviation.
The variance of factory $A$ is 100 and the variance of factory $B$ is 81 .
Now, $S D$ of factory $A=\sqrt{100}=10$
And, $S D$ of factory $B=\sqrt{81}=9$
Therefore,
The $\mathrm{CV}$ of factory $\mathrm{A}=\frac{10}{5300} \times 100=0.189$
The $C V$ of factory $B=\frac{9}{5300} \times 100=0.169$
Here, the CV of factory A is greater than the CV of factory B.
Hence, factory A has more variation.
