Question:
The foot of the perpendicular drawn from the point $(4,2,3)$ to the line joining the points $(1,-2,3)$ and $(1,1,0)$ lies on the plane:
Correct Option: 1
Solution:
Equation of line through points $(1,-2,3)$ and $(1,1,0)$ is
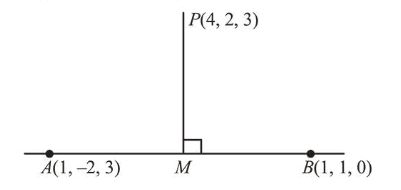
$\frac{x-1}{0}=\frac{y-1}{-3}=\frac{z-0}{3-0} \quad(=\lambda$ say $)$
$\therefore M(1,-\lambda+1, \lambda)$
Direction ratios of $\mathrm{PM}=[-3,-\lambda-1, \lambda-3]$
$\because P M \perp A B$
$\therefore(-3) \cdot 0+(-1-\lambda)(-1)+(\lambda-3) \cdot 1=0$
$\therefore \lambda=1$
$\therefore$ Foot of perpendicular $=(1,0,1)$
This point satisfy the plane $2 x+y-z=1$.
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
