The initial velocity $v_{i}$ required to project a body vertically upward from the surface of the earth to reach a height of $10 \mathrm{R}$, where $\mathrm{R}$ is the
radius of the earth, may be described in terms of escape velocity $v_{c}$ such that $v_{i}=\sqrt{\frac{x}{y}} \times v_{e}$. The value of $x$ will be
(10)
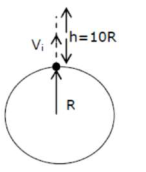
Here $\mathrm{R}=$ radius of the earth
From energy conservation
$\frac{-\mathrm{Gm}_{\mathrm{m}} \mathrm{m}}{\mathrm{R}}+\frac{1}{2} \mathrm{mv}_{\mathrm{i}}^{2}=\frac{-\mathrm{Gm}_{\mathrm{m}} \mathrm{m}}{11 \mathrm{R}}+0$
$\frac{1}{2} m v_{i}^{2}=\frac{10}{11} \frac{G m_{e} m}{R}$
$V_{i}=\sqrt{\frac{20}{11} \frac{\mathrm{Gm}_{e}}{\mathrm{R}}}$
$V_{i}=\sqrt{\frac{10}{11}} v_{e} \quad\left\{\because\right.$ escape velocity $\left.v_{e}=\sqrt{\frac{2 G m_{e}}{R}}\right\}$
Then the value of $x=10$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.