The perimeter of the sector OAB shown in the following figure, is

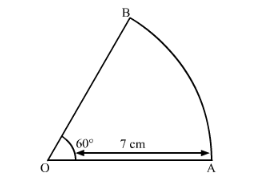
We know that perimeter of a sector of radius $l=2 r+\frac{\theta}{360} \times 2 \pi r$.....(1)

We have given sector angle and radius of the sector and we are asked to find perimeter of the sector OAB.

Therefore, substituting the corresponding values of the sector angle and radius in equation (1) we get,

Perimeter $=2 \times 7+\frac{60}{360} \times 2 \pi \times 7$.......(2)

We will simplify equation (2) as shown below,

Perimeter $=2 \times 7+\frac{1}{6} \times 2 \pi \times 7$

Substituting $\pi=\frac{22}{7}$ we get,

Perimeter $=2 \times 7+\frac{1}{6} \times 2 \times \frac{22}{7} \times 7$

$\therefore$ Perimeter $=2 \times 7+\frac{1}{6} \times 2 \times 22$

$\therefore$ Perimeter $=2 \times 7+\frac{1}{3} \times 22$

$\therefore$ Perimeter $=2 \times 7+\frac{22}{3}$

$\therefore$ Perimeter $=14+\frac{22}{3}$

Now we will make the denominator same.

$\therefore$ Perimeter $=\frac{42}{3}+\frac{22}{3}$

$\therefore$ Perimeter $=\frac{42+22}{3}$

$\therefore$ Perimeter $=\frac{64}{3}$

Therefore, perimeter of the sector is $\frac{64}{3} \mathrm{~cm}$.