Question:
The sides BC, CA and AB of ∆ABC have been produced to D, E and F, respectively. ∠BAE + ∠CBF + ∠ACD = ?
(a) 240°
(b) 300°
(c) 320°
(d) 360°
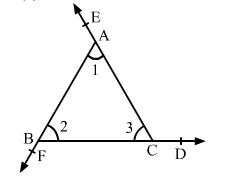
Solution:
(d) 360°
We have :
$\angle 1+\angle B A E=180^{\circ} \ldots($ i $)$
$\angle 2+\angle C B F=180^{\circ} \quad \ldots($ ii $)$ and
$\angle 3+\angle A C D=180^{\circ} \quad \ldots($ iii $)$
Adding $(i),(i i)$ and $(i i i)$, we get:
$(\angle 1+\angle 2+\angle 3)+(\angle B A E+\angle C B F+\angle A C D)=540^{\circ}$
$\Rightarrow 180^{\circ}+\angle B A E+\angle C B F+\angle A C D=540^{\circ} \quad\left[\because \angle 1+\angle 2+\angle 3=180^{\circ}\right]$
$\Rightarrow \angle B A E+\angle C B F+\angle A C D=360^{\circ}$
