Question:
The value of $\sqrt{6+\sqrt{6+\sqrt{6+}} \ldots .}$ is
(a) 4
(b) 3
(c) −2
(d) 3.5
Solution:
Let $x=\sqrt{6+\sqrt{6+\sqrt{6+\sqrt{6+} \ldots . .}}}$
Squaring both sides we get
$x^{2}=6+\sqrt{6+\sqrt{6+\sqrt{6+\sqrt{6+\ldots . . .}}}}$
$x^{2}=6+x$
$x^{2}-x-6=0$
$x^{2}-3 x+2 x-6=0$
$x(x-3)+2(x-3)=0$
$(x-3)(x+2)=0$
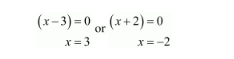
The value of x cannot be negative.
Thus, the value of x = 3
Therefore, the correct answer is $(b)$
