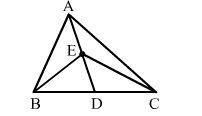
The vertex A of ∆ABC is joined to a point D on BC.

(a) $\frac{1}{2} \operatorname{ar}(\Delta A B C)$

Since E is the midpoint of AD, BE is a median of ∆ABD.
We know that a median of a triangle divides it into two triangles of equal areas.

i.e., $\operatorname{ar}(\Delta B E D)=\frac{1}{2} \times \operatorname{ar}(\triangle A B D)$       ...(i)

Since E is the midpoint of AD, CE is a median of ∆ADC.
We know that a median of a triangle divides it into two triangles of equal areas.

i.e., $\operatorname{ar}(\triangle C E D)=\frac{1}{2} \times \operatorname{ar}(\triangle A D C)$      ...(ii)

Adding (i) and (ii), we have:

$\operatorname{ar}(\Delta B E D)+\operatorname{ar}(\Delta C E D)=\frac{1}{2} \times \operatorname{ar}(\Delta A B D)+\frac{1}{2} \times \operatorname{ar}(\Delta A D C)$

$\Rightarrow \operatorname{ar}(\Delta B E C)=\frac{1}{2}(\Delta A B D+A D C)=\frac{1}{2} \Delta A B C$