Two capacitors of capacitances $\mathrm{C}$ and $2 \mathrm{C}$ are charged to potential differences $\mathrm{V}$ and $2 \mathrm{~V}$, respectively. These are then connected in parallel in such a manner that the positive terminal of one is connected to the negative terminal of the other. The final energy of this configuration is :
Correct Option: , 2
(b) When capacitors $C$ and $2 C$ capacitance are charged to $\mathrm{V}$ and $2 \mathrm{~V}$ respectively.
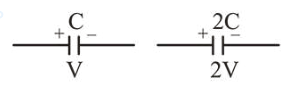
$Q_{1}=C V \quad Q_{2}=2 C \times 2 V=4 C V$
When connected in parallel
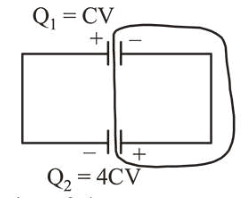
By conservation of charge
$4 C V-C V=(C+2 C) V_{\text {common }}$
$V_{\text {common }}=\frac{3 C V}{3 C}=V$
Therefore final energy of this configuration,
$U_{f}=\left(\frac{1}{2} C V^{2}+\frac{1}{2} \times 2 C V^{2}\right)=\frac{3}{2} C V^{2}$
