Question:
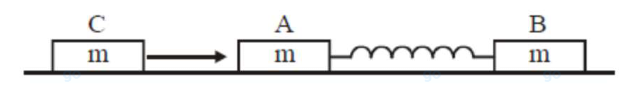
Two identical blocks $A$ and $B$ each of mass $m$
resting on the smooth horizontal floor are connected by a light spring of natural length $L$ and spring constant $\mathrm{K}$. A third block $\mathrm{C}$ of mass $\mathrm{m}$ moving with a speed $\mathrm{v}$ along the line joining $\mathrm{A}$ and $\mathrm{B}$ collides with $\mathrm{A}$. The maximum compression in the spring is
Correct Option: 1
Solution:
(1)
$\mathrm{C}$ comes to rest
$V_{c m}$ of $A \& B=\frac{v}{2}$
$\Rightarrow \frac{1}{2}$ is $\mathrm{v}_{\mathrm{ret}}^{2}=\frac{1}{2} \mathrm{kx}^{2}$\
$x=\sqrt{\frac{\mu \times v^{2}}{k}}=\sqrt{\frac{m}{2 k}} v$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.