Question:
Two lines AB and CD intersect each other at a point O such that ∠AOC : ∠AOD = 5 : 7. Find all the angles.
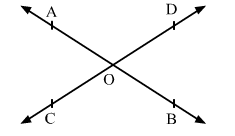
Solution:
Let $\angle \mathrm{AOC}=5 k$ and $\angle \mathrm{AOD}=7 k$, where $k$ is some constant.
Here, $\angle A O C$ and $\angle A O D$ form a linear pair.
$\therefore \angle \mathrm{AOC}+\angle \mathrm{AOD}=180^{\circ}$
$\Rightarrow 5 k+7 k=180^{\circ}$
$\Rightarrow 12 k=180^{\circ}$
$\Rightarrow k=15^{\circ}$
$\therefore \angle \mathrm{AOC}=5 \mathrm{k}=5 \times 15^{\circ}=75^{\circ}$
$\angle \mathrm{AOD}=7 k=7 \times 15^{\circ}=105^{\circ}$
Now, $\angle B O D=\angle A O C=75^{\circ}$ (Vertically opposite angles)
$\angle B O C=\angle A O D=105^{\circ}$ (Vertically opposite angles)
