Two particles of equal mass $m$ have respective initial velocities $u \hat{i}$ and $u\left(\frac{\hat{i}+\hat{j}}{2}\right)$. They collide completely inelastically. The energy lost in the process is:
Correct Option: , 2
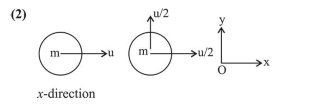
$m u+\frac{m u}{2}=2 m v_{x}^{\prime} \Rightarrow V_{x}^{\prime}=\frac{3 u}{4}$
$\mathrm{y}$-direction $0+\frac{m u}{2}=2 m v_{y}^{\prime} \Rightarrow v_{y}^{\prime}=\frac{u}{4}$
$K \cdot E_{\cdot i}=\frac{1}{2} m u^{2}+\frac{1}{2} m\left[\left(\frac{u}{2}\right)^{2}+\left(\frac{u}{2}\right)^{2}\right]$
$=\frac{1}{2} m u^{2}+\frac{m u^{2}}{4}=\frac{3 m u^{2}}{4}$
$K . \mathrm{E}_{\cdot f}=\frac{1}{2}(2 m)\left(v_{x}^{\prime}\right)^{2}+\frac{1}{2}(2 m)\left(v_{y}^{\prime}\right)^{2}$
$=\frac{1}{2} 2 m\left[\left(\frac{3 u}{4}\right)^{2}+\left(\frac{u}{4}\right)^{2}\right]=\frac{5}{8} m u^{2}$
$\therefore$ Loss in $K E=K E_{f}-K E_{i}$
$=m u^{2}\left(\frac{6}{8}-\frac{5}{8}\right)=\frac{m u^{2}}{8}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.