Question:
Two vertical poles $\mathrm{AB}=15 \mathrm{~m}$ and $\mathrm{CD}=10 \mathrm{~m}$ are standing apart on a horizontal ground with points $\mathrm{A}$ and $\mathrm{C}$ on the ground. If $\mathrm{P}$ is the point of intersection of $\mathrm{BC}$ and $\mathrm{AD}$, then the height of $P$ (in $m$ ) above the line $A C$ is :
Correct Option: , 4
Solution:
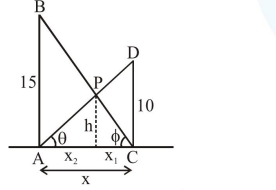
$\tan \theta=\frac{10}{\mathrm{x}}=\frac{\mathrm{h}}{\mathrm{x}_{2}} \Rightarrow \mathrm{x}_{2}=\frac{\mathrm{hx}}{10}$
$\tan \phi=\frac{15}{\mathrm{x}}=\frac{\mathrm{h}}{\mathrm{x}_{1}} \Rightarrow \mathrm{x}_{1}=\frac{\mathrm{hx}}{15}$
Now, $x_{1}+x_{2}=x=\frac{h x}{15}+\frac{h x}{10}$
$\Rightarrow 1=\frac{\mathrm{h}}{10}+\frac{\mathrm{h}}{15} \Rightarrow \mathrm{h}=6$
