At time $t=0$ magnetic field of 1000 Gauss is passing perpendicularly through the area defined by the closed loop shown in the figure. If the magnetic field reduces linearly to 500 Gauss, in the next $5 \mathrm{~s}$, then induced EMF in the loop is:
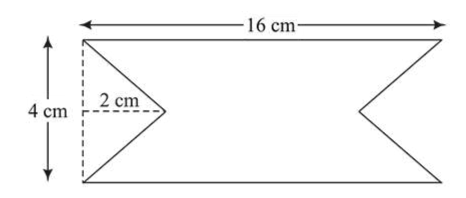
Correct Option: 1
(1) According to question, $d B=1000-500=500$ gauss
$=500 \times 10^{-4} \mathrm{~T}$
Time $d t=5 \mathrm{~s}$
Using faraday law
Induced $E M F, e=\left|-\frac{d \phi}{d t}\right|=\left|A \frac{d B}{d t}\right|$
$\frac{d B}{d t}=\frac{1000-500}{5} \times 10^{-4}=10^{-2} \mathrm{~T} / \mathrm{sec}$
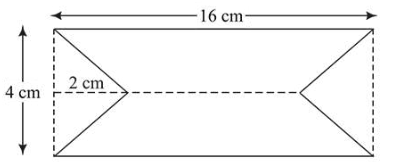
Area, $A=$ ar of $\square-2$ ar of $\Delta=(16 \times 4-2 \times$ Area of triangle) $\mathrm{cm}^{2}$
$=\left(64-2 \times \frac{1}{2} \times 2 \times 4\right) \mathrm{cm}^{2}$
$=56 \times 10^{-4} \mathrm{~m}^{2}$
$\therefore \varepsilon_{\text {induced }}=\left|A \frac{d B}{d t}\right|=56 \times 10^{-4} \times 10^{-2}=56 \times 10^{-6} \mathrm{~V}=56 \mu \mathrm{V}$
