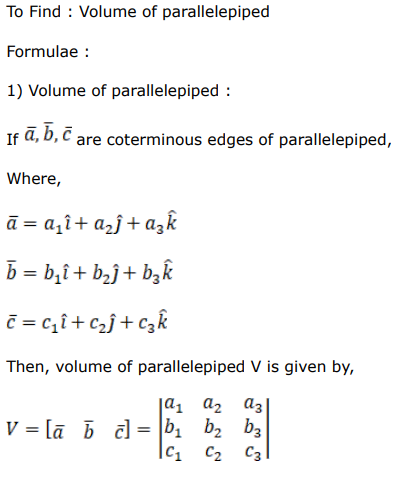
Find the volume of the parallelepiped whose conterminous edges are represented by the vectors+
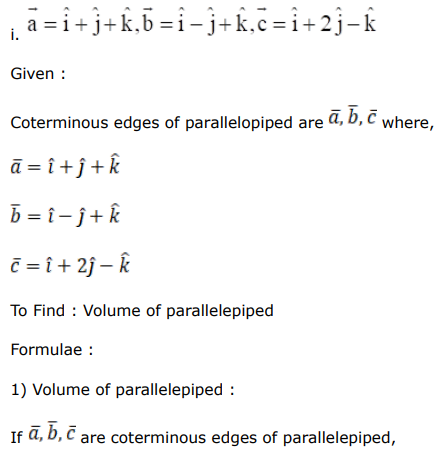
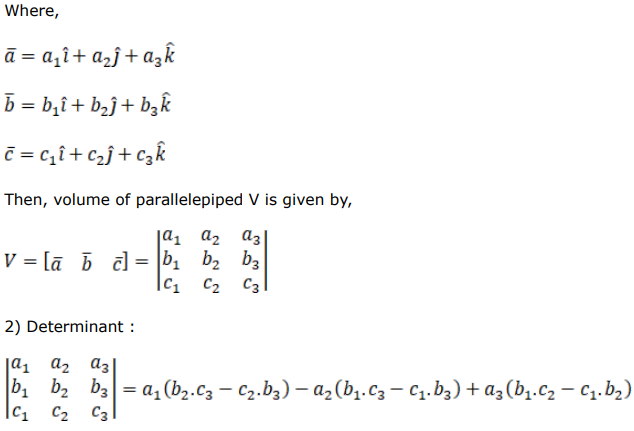
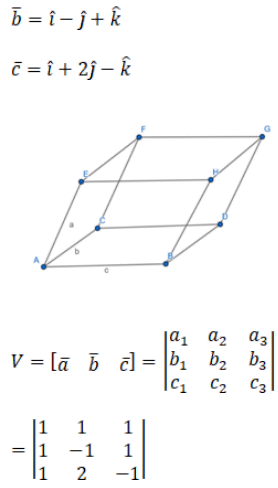
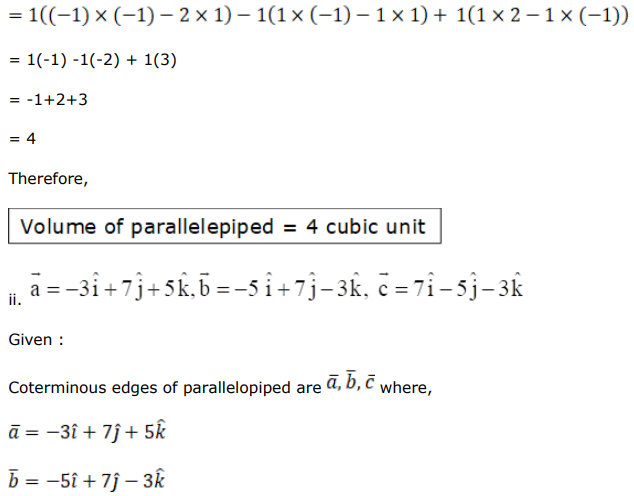
i. $\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}+\hat{\mathrm{j}}+\hat{\mathrm{k}}, \overrightarrow{\mathrm{b}}=\hat{\mathrm{i}}-\hat{\mathrm{j}}+\hat{\mathrm{k}}, \overrightarrow{\mathrm{c}}=\hat{\mathrm{i}}+2 \hat{\mathrm{j}}-\hat{\mathrm{k}}$
ii. $\overrightarrow{\mathrm{a}}=-3 \hat{\mathrm{i}}+7 \hat{\mathrm{j}}+5 \hat{\mathrm{k}}, \overrightarrow{\mathrm{b}}=-5 \hat{\mathrm{i}}+7 \hat{\mathrm{j}}-3 \hat{\mathrm{k}}, \overrightarrow{\mathrm{c}}=7 \hat{\mathrm{i}}-5 \hat{\mathrm{j}}-3 \hat{\mathrm{k}}$
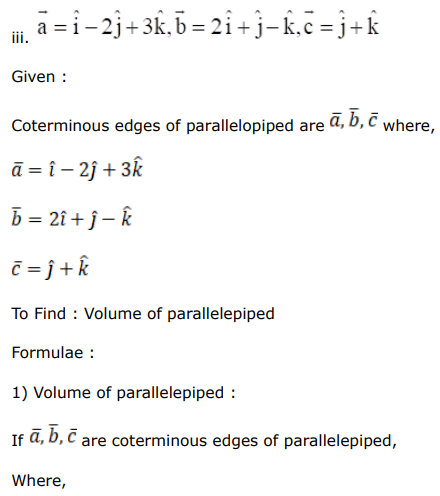
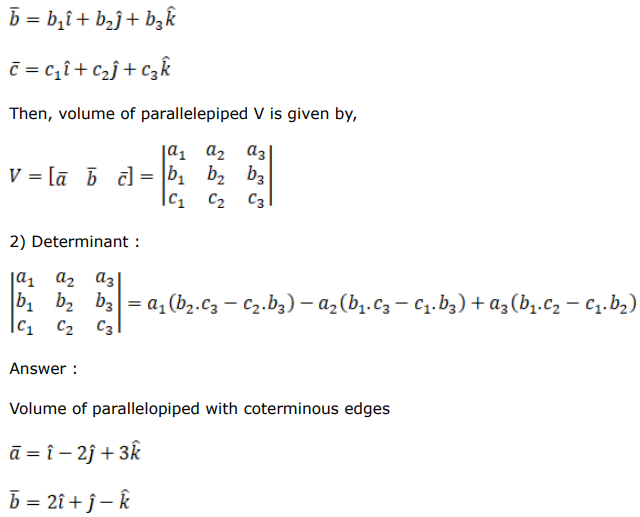
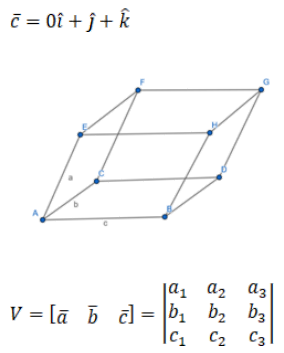
iii. $\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}-2 \hat{\mathrm{j}}+3 \hat{\mathrm{k}}, \overrightarrow{\mathrm{b}}=2 \hat{\mathrm{i}}+\hat{\mathrm{j}}-\hat{\mathrm{k}}, \overrightarrow{\mathrm{c}}=\hat{\mathrm{j}}+\hat{\mathrm{k}}$
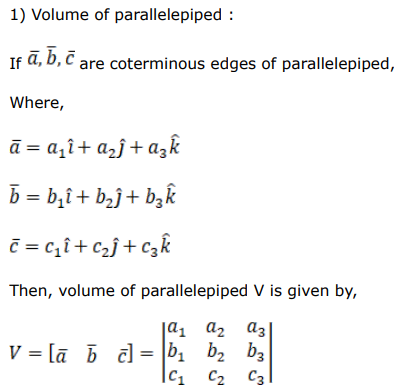
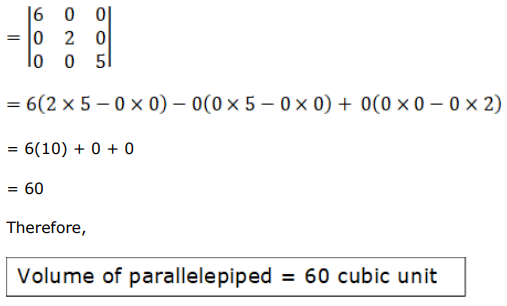
iv. $\bar{a}=6 \hat{l}, \bar{b}=2 \hat{\jmath}, \bar{c}=5 \hat{k}$

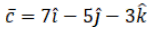

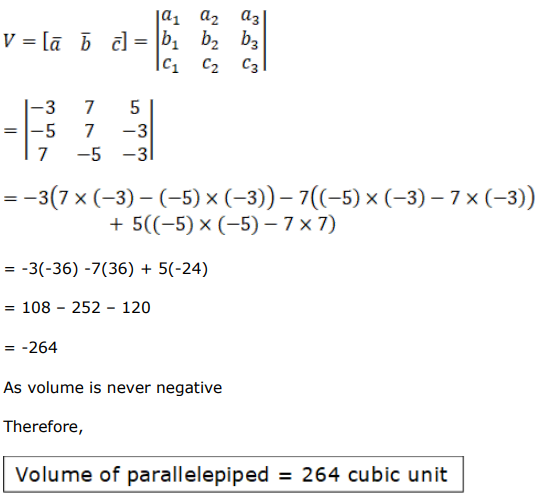

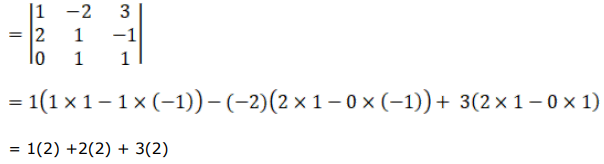
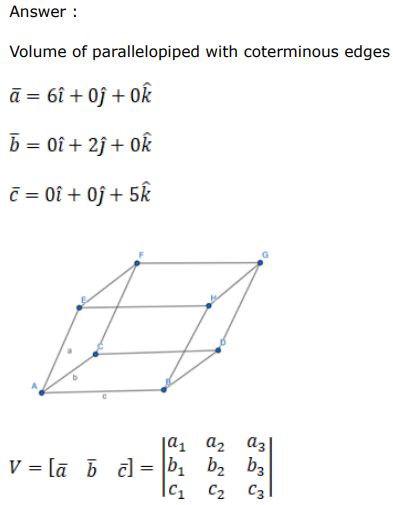
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.
