If E, F, G and H are respectively the mid-points of the sides of a parallelogram

Solution:



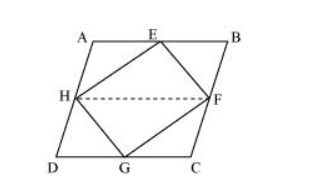
Let us join HF.

In parallelogram ABCD,

AD = BC and AD || BC (Opposite sides of a parallelogram are equal and parallel)

AB = CD (Opposite sides of a parallelogram are equal)

$\Rightarrow \frac{1}{2} \mathrm{AD}=\frac{1}{2} \mathrm{BC}$ and $\mathrm{AH} \| \mathrm{BF}$

$\Rightarrow \mathrm{AH}=\mathrm{BF}$ and $\mathrm{AH} \| \mathrm{BF}(\because \mathrm{H}$ and $\mathrm{F}$ are the mid-points of $\mathrm{AD}$ and $\mathrm{BC})$

Therefore, $\mathrm{ABFH}$ is a parallelogram.

Since $\triangle \mathrm{HEF}$ and parallelogram $\mathrm{ABFH}$ are on the same base $\mathrm{HF}$ and between the same parallel lines $\mathrm{AB}$ and $\mathrm{HF}$,

$\therefore$ Area $(\Delta \mathrm{HEF})=\frac{1}{2}$ Area $(\mathrm{ABFH}) \ldots$(1)

Similarly, it can be proved that

Area $(\Delta H G F)=\frac{1}{2}$ Area $(H D C F) \ldots$(2)

On adding equations (1) and (2), we obtain

Area $(\Delta \mathrm{HEF})+$ Area $(\Delta \mathrm{HGF})=\frac{1}{2}$ Area $(\mathrm{ABFH})+\frac{1}{2}$ Area $(\mathrm{HDCF})$

$=\frac{1}{2}[$ Area $(\mathrm{ABFH})+$ Area $(\mathrm{HDCF})]$

$\Rightarrow$ Area $(\mathrm{EFGH})=\frac{1}{2}$ Area $(\mathrm{ABCD})$