Question:
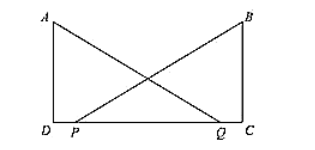
In figure, AD ⊥ CD and CB ⊥ CD. If AQ = BP and DP = CQ, prove that ∠DAQ = ∠CBP.
Solution:
Given in the figure, AD ⊥ CD and CB ⊥ CD.
And AQ = BP and DP = CQ,
To prove that ∠DAQ = ∠CBP
Given that DP = QC
Add PQ on both sides
DP + PQ = PQ + QC
DQ = PC ... (i)
Now, consider triangle DAQ and CBP,
We have
∠ADQ = ∠BCP = 90° [given] AQ = BP [given]
And DQ = PC [From (i)]
So, by RHS congruence criterion, we have
ΔDAQ ≅ ΔCBP
Now,
∠DAQ = ∠CBP [Corresponding parts of congruent triangles are equal]
Hence proved
