Question:
Show that the solution set of the following system of linear inequalities is an unbounded region: $2 x+y \geq 8, x+2 y \geq 10, x \geq 0, y \geq 0$.
Solution:
We have:
$2 x+y \geq 8$ ...(i)
$x+2 y \geq 10$ ...(ii)
$x \geq 0$ ....(iii)
$y \geq 0$ ...(iv)
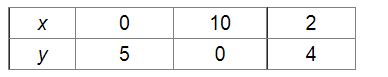
As, the solutions of the equation 2x + y = 8 are:

As, the solutions of the equation x + 2y = 10 are:
Now, the graph represented by the inequalities (i), (ii), (iii) and (iv) is as follows:
Since, the common shaded region is the solution set of the given set of inequalities.
So, the solution set of the given linear inequalities is an unbounded region.
