Solve this following

Question:

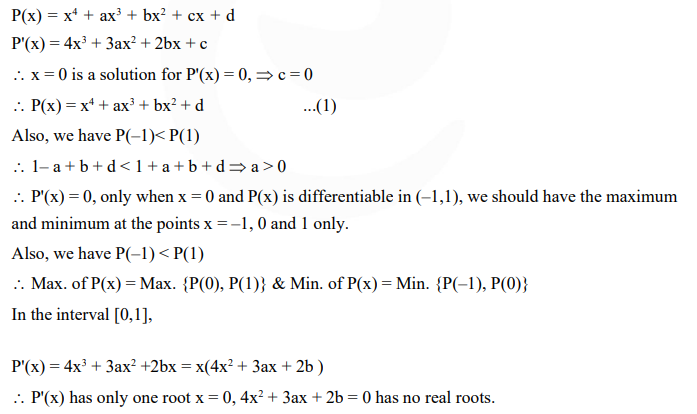
Given $\mathrm{P}(\mathrm{x})=\mathrm{x}^{4}+\mathrm{ax}^{3}+b \mathrm{x}^{2}+\mathrm{cx}+\mathrm{d}$ such that $\mathrm{x}=0$ is the only real root of $\mathrm{P}^{\prime}(\mathrm{x})=0$. If $\mathrm{P}(-1)<\mathrm{P}(1)$, then in the interval $[-1,1]$ :-

  1. $P(-1)$ is the minimum but $P(1)$ is not the maximum of $P$.

  2. Neither $\mathrm{P}(-1)$ is the minimum nor $\mathrm{P}(1)$ is the maximum of $\mathrm{P}$

  3. $\mathrm{P}(-1)$ is the minimum and $\mathrm{P}(1)$ is the maximum of $\mathrm{P}$

  4. $P(-1)$ is not minimum but $P(1)$ is the maximum of $P$

Correct Option: , 4

Solution:

 

Leave a comment

eSaral Gurukul

NEET

JEE Main

JEE Advanced

Our Telegram Channel

All Study Material