NCERT Solutions for Class 9 Maths chapter 2 Polynomials PDF

Hey, are you a class 9 student and looking for ways to download NCERT Solutions for Class 9 Maths chapter 2 Polynomials PDF? If yes. Then read this post till the end.

In this article, we have listed NCERT Solutions for Class 9 Maths chapter 2 Polynomials in PDF that is prepared by Kota’s top IITian’s Faculties by keeping Simplicity in mind.

If you want to learn and understand class 9 Maths chapter 2 "Polynomials" in an easy way then you can use these solutions PDF.

NCERT Solutions helps students to Practice important concepts of subjects easily. Class 9 Maths solutions provide detailed explanations of all the NCERT exercise questions that students can use to clear their doubts instantly.

If you want to score high in your class 9 Maths Exam then it is very important for you to have a good knowledge of all the important topics, so to learn and practice those topics you can use eSaral NCERT Solutions.

In this article, we have listed NCERT Solutions for Class 9 Maths chapter 2 Polynomials PDF that you can download to start your preparations anytime.

So, without wasting more time Let’s start.

Download NCERT Solutions for Class 9 Maths chapter 2 Polynomials PDF"

Download The PDF of NCERT Solutions for Class 9 Maths Chapter 2 "Polynomials"

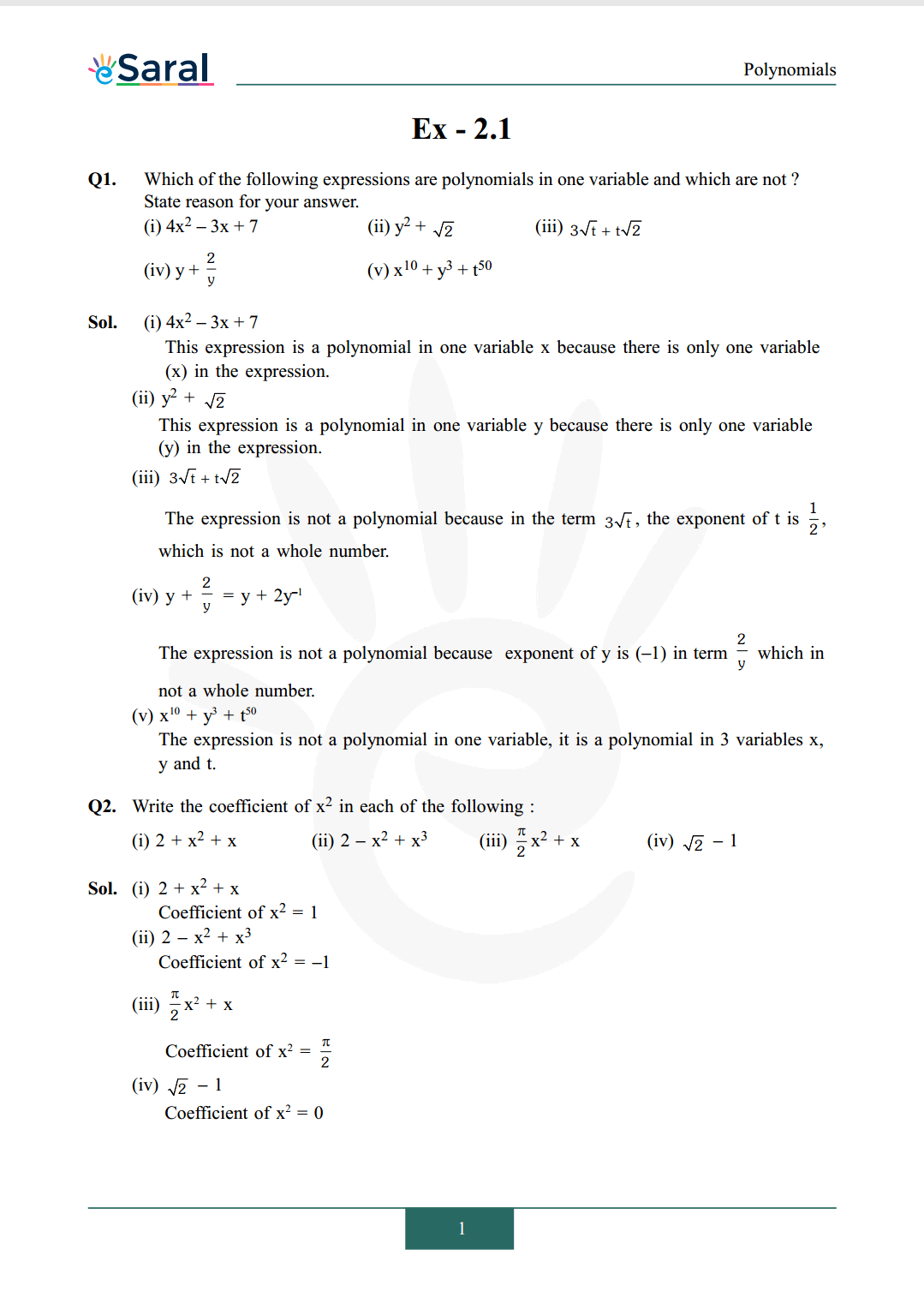
NCERT Solutions for Class 9 Maths chapter 2 Ex - 2.1

Q1. Which of the following expressions are polynomials in one variable and which are not ? State reason for your answer.
(i) $4 x^{2}-3 x+7$
(ii) $\mathrm{y}^{2}+\sqrt{2}$
(iii) $3 \sqrt{t}+t \sqrt{2}$
(iv) $\mathrm{y}+\frac{2}{\mathrm{y}}$
(v) $x^{10}+y^{3}+t^{50}$

Sol. (i) $4 x^{2}-3 x+7$
This expression is a polynomial in one variable $x$ because there is only one variable (x) in the expression.
(ii) $y^{2}+\sqrt{2}$
This expression is a polynomial in one variable y because there is only one variable (y) in the expression.
(iii) $3 \sqrt{t}+t \sqrt{2}$
The expression is not a polynomial because in the term $3 \sqrt{\mathrm{t}}$, the exponent of $\mathrm{t}$ is $\frac{1}{2}$,which is not a whole number.
(iv) $y+\frac{2}{y}=y+2 y^{-1}$
The expression is not a polynomial because exponent of $y$ is $(-1)$ in term $\frac{2}{y}$ which in
not a whole number.
(v) $x^{10}+y^{3}+t^{50}$
The expression is not a polynomial in one variable, it is a polynomial in 3 variables $\mathrm{x}$, $\mathrm{y}$ and $\mathrm{t}$.

Q2. Write the coefficient of $\mathrm{x}^{2}$ in each of the following :
(i) $2+x^{2}+x$
(ii) $2-x^{2}+x^{3}$
(iii) $\frac{\pi}{2} \mathrm{x}^{2}+\mathrm{x}$
(iv) $\sqrt{2}-1$

Sol. (i) $2+\mathrm{x}^{2}+\mathrm{x}$
Coefficient of $x^{2}=1$
(ii) $2-\mathrm{x}^{2}+\mathrm{x}^{3}$
Coefficient of $x^{2}=-1$
(iii) $\frac{\pi}{2} x^{2}+x$
Coefficient of $\mathrm{x}^{2}=\frac{\pi}{2}$
(iv) $\sqrt{2}-1$
Coefficient of $x^{2}=0$

Q3. Give one example each of a binomial of degree 35 and of a monomial of degree 100 .

Sol. One example of a binomial of degree 35 is $3 \mathrm{x}^{35}-4 .$
One example of monomial of degree 100 is $5 \mathrm{x}^{100}$.

Q4. Write the degree of each of the following polynomials:
(i) $5 x^{3}+4 x^{2}+7 x$
(ii) $4-\mathrm{y}^{2}$
(iii) $5 \mathrm{t}-\sqrt{7}$
(iv) 3

Sol. (i) $5 x^{3}+4 x^{2}+7 x$
Term with the highest power of $x=5 x^{3}$
Exponent of $x$ in this term $=3$
$\therefore$ Degree of this polynomial $=3$.
(ii) $4-\mathrm{y}^{2}$
Term with the highest power of $\mathrm{y}=-\mathrm{y}^{2}$
Exponent of $\mathrm{y}$ in this term $=2$
$\therefore$ Degree of this polynomial $=2$
(iii) $5 \mathrm{t}-\sqrt{7}$
Term with highest power of $\mathrm{t}=5 \mathrm{t}$.
Exponent of $t$ in this term $=1$
$\therefore$ Degree of this polynomial $=1$.
(iv) 3
This is a constant which is non-zero
So, degree of this polynomial $=0$

Q5. Classify the following as linear, quadratic and cubic polynomials :
(i) $x^{2}+x$
(ii) $x-x^{3}$
(iii) $\mathrm{y}+\mathrm{y}^{2}+4$
(iv) $1+x \quad$
(v) $3 \mathrm{t}$
(vi) $r^{2}$
(vii) $7 \mathrm{x}^{2}$

Sol. (i) Quadratic
(ii) Cubic
(iii) Quadratic
(iv) Linear
(v) Linear
(vi) Quadratic
(vii) Quadratic

NCERT Solutions for Class 9 Maths chapter 2 Ex - 2.2

Q1. Find the value of the polynomial $5 x-4 x^{2}+3$ at
(i) $x=0$
(ii) $x=-1$
(iii) $x=2$

Sol. Let $f(x)=5 x-4 x^{2}+3$
(i) Value of $\mathrm{f}(\mathrm{x})$ at $\mathrm{x}=0=\mathrm{f}(0)$
$=5(0)-4(0)^{2}+3=3$
(ii) Value of $\mathrm{f}(\mathrm{x})$ at $\mathrm{x}=-1=\mathrm{f}(-1)$
$=5(-1)-4(-1)^{2}+3=-5-4+3=-6$
(iii) Value of $\mathrm{f}(\mathrm{x})$ at $\mathrm{x}=2=\mathrm{f}(2)$
$=5(2)-4(2)^{2}+3$
$=10-16+3=-3$

Q2. Find $p(0), p(1), p(2)$, for each of the following polynomials :
(i) $p(y)=y^{2}-y+1$
(ii) $p(t)=2+t+2 t^{2}-t^{3}$
(iii) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{3}$
(iv) $\mathrm{p}(\mathrm{x})=(\mathrm{x}-1)(\mathrm{x}+1)$

Sol. (i) $p(y)=y^{2}-y+1$
$\therefore \mathrm{p}(0)=(0)^{2}-(0)+1=1$
$\mathrm{p}(1)=(1)^{2}-(1)+1=1$
$\mathrm{p}(2)=(2)^{2}-(2)+1=4-2+1=3$
(ii) $p(t)=2+t+2 t^{2}-t^{3}$
$\mathrm{p}(0)=2+0+2(0)^{2}-(0)^{3}=2$
$p(1)=2+1+2(1)^{2}-(1)^{3}=2+1+2-1=4$
$\mathrm{p}(2)=2+2+2(2)^{2}-(2)^{3}=2+2+8-8=4$
(iii) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{3}$
$\mathrm{p}(0)=(0)^{3}=0$
$p(1)=(1)^{3}=1$
$p(2)=(2)^{3}=8$
(iv) $p(x)=(x-1)(x+1)$
$p(0)=(0-1)(0+1)=(-1)(1)=-1$
$\mathrm{p}(1)=(1-1)(1+1)=0(2)=0$
$p(2)=(2-1)(2+1)=(1)(3)=3$

Q3. Verify whether the following are zeroes of the polynomial, indicated against them,
(i) $\mathrm{p}(\mathrm{x})=3 \mathrm{x}+1, \mathrm{x}=-\frac{1}{3}$
(ii) $\mathrm{p}(\mathrm{x})=5 \mathrm{x}-\pi, \mathrm{x}=\frac{4}{5}$
(iii) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{2}-1, \mathrm{x}=1,-1$
(iv) $p(x)=(x+1)(x-2), x=-1,2$
(v) $p(x)=x^{2}, x=0$
(vi) $\mathrm{p}(\mathrm{x})=\ell \mathrm{x}+\mathrm{m}, \mathrm{x}=-\frac{\mathrm{m}}{\ell}$
(vii) $\mathrm{p}(\mathrm{x})=3 \mathrm{x}^{2}-1, \mathrm{x}=-\frac{1}{\sqrt{3}}, \frac{2}{\sqrt{3}}$
(viii) $\mathrm{p}(\mathrm{x})=2 \mathrm{x}+1, \mathrm{x}=\frac{1}{2}$

Sol. (i) $\mathrm{p}(\mathrm{x})=3 \mathrm{x}+1, \mathrm{x}=-\frac{1}{3}$
$p\left(-\frac{1}{3}\right)=3\left(-\frac{1}{3}\right)+1=-1+1=0$
$\therefore-\frac{1}{3}$ is a zero of $\mathrm{p}(\mathrm{x})$.
(ii) $\mathrm{p}(\mathrm{x})=5 \mathrm{x}-\pi, \mathrm{x}=\frac{4}{5}$
$\mathrm{p}\left(\frac{4}{5}\right)=5\left(\frac{4}{5}\right)-\pi=4-\pi \neq 0$
$\therefore \frac{4}{5}$ is not a zero of $\mathrm{p}(\mathrm{x})$
(iii) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{2}-1, \mathrm{x}=1,-1$
$\mathrm{p}(1)=(1)^{2}-1=1-1=0$
$p(-1)=(-1)^{2}-1=1-1=0$
$\therefore 1,-1$ are zero's of $\mathrm{p}(\mathrm{x})$.
(iv) $p(x)=(x+1)(x-2), \quad x=-1,2$
$p(-1)=(-1+1)(-1-2)=(0)(-3)=0$
$\mathrm{p}(2)=(2+1)(2-2)=(3)(0)=0$
$\therefore-1,2$ are zero's of $\mathrm{p}(\mathrm{x})$
(v) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{2}, \mathrm{x}=0$
$\mathrm{p}(0)=0$
$\therefore 0$ is a zero of $\mathrm{p}(\mathrm{x})$
(vi) $\mathrm{p}(\mathrm{x})=\ell \mathrm{x}=\mathrm{m}, \mathrm{x}=\frac{-\mathrm{m}}{\ell}$
$\mathrm{p}\left(\frac{-\mathrm{m}}{\ell}\right)=\ell\left(\frac{-\mathrm{m}}{\ell}\right)+\mathrm{m}=-\mathrm{m}+\mathrm{m}=0$
$\therefore \frac{-\mathrm{m}}{\ell}$ is a zero of $\mathrm{p}(\mathrm{x})$.
(vii) $\mathrm{p}(\mathrm{x})=3 \mathrm{x}^{2}-1, \mathrm{x}=-\frac{1}{\sqrt{3}}, \frac{2}{\sqrt{3}}$
$\mathrm{p}\left(-\frac{1}{\sqrt{3}}\right)=3\left(-\frac{1}{\sqrt{3}}\right)^{2}-1=3\left(\frac{1}{3}\right)-1$
$=1-1=0$
$\mathrm{p}\left(\frac{2}{\sqrt{3}}\right)=3\left(\frac{2}{\sqrt{3}}\right)^{2}-1=3\left(\frac{4}{3}\right)-1$
$=4-1=3 \neq 0$
So, $-\frac{1}{\sqrt{3}}$ is a zero of $\mathrm{p}(\mathrm{x})$ and $\frac{2}{\sqrt{3}}$ is not a zero of $\mathrm{p}(\mathrm{x})$.
(viii) $\mathrm{p}(\mathrm{x})=2 \mathrm{x}+1, \mathrm{x}=\frac{1}{2}$
$\mathrm{p}\left(\frac{1}{2}\right)=2\left(\frac{1}{2}\right)+1=1+1=2 \neq 0$
$\therefore \frac{1}{2}$ is not a zero of $\mathrm{p}(\mathrm{x})$.

Q4. Find the zero of the polynomial in each of the following cases:
(i) $\mathrm{p}(\mathrm{x})=\mathrm{x}+5$
(ii) $\mathrm{p}(\mathrm{x})=\mathrm{x}-5$
(iii) $\mathrm{p}(\mathrm{x})=2 \mathrm{x}+5$
(iv) $\mathrm{p}(\mathrm{x})=3 \mathrm{x}-2$
(v) $\mathrm{p}(\mathrm{x})=3 \mathrm{x}$
(vi) $\mathrm{p}(\mathrm{x})=\mathrm{ax}, \mathrm{a} \neq 0$
(vii) $\mathrm{p}(\mathrm{x})=\mathrm{cx}+\mathrm{d}, \mathrm{c} \neq 0, \mathrm{c}, \mathrm{d}$ are real numbers.

Sol. (i) $\mathrm{p}(\mathrm{x})=\mathrm{x}+5$
$p(x)=0$
$\Rightarrow x+5=0 \Rightarrow x=-5$
$\therefore-5$ is zero of the polynomial $\mathrm{p}(\mathrm{x})$.
(ii) $\mathrm{p}(\mathrm{x})=\mathrm{x}-5$
$p(x)=0$
$x-5=0$
or $x=5$
$\therefore 5$ is zero of polynomial $\mathrm{p}(\mathrm{x})$.
(iii) $\mathrm{p}(\mathrm{x})=2 \mathrm{x}+5$
$p(x)=0$
$2 x+5=0$
$2 x=-5$
$\Rightarrow \mathrm{x}=-\frac{5}{2}$
$\therefore-\frac{5}{2}$ is zero of polynomial $\mathrm{p}(\mathrm{x})$.
(iv) $p(x)=3 x-2$
$\mathrm{p}(\mathrm{x})=0 \Rightarrow 3 \mathrm{x}-2=0$
or $x=\frac{2}{3}$
$\therefore \frac{2}{3}$ is zero of polynomial $\mathrm{p}(\mathrm{x})$
(v) $\mathrm{p}(\mathrm{x})=3 \mathrm{x}$
$p(x)=0 \Rightarrow 3 x=0$
or $\mathbf{x}=0$
$\therefore 0$ is zero of polynomial $\mathrm{p}(\mathrm{x})$.
(vi) $\mathrm{p}(\mathrm{x})=\mathrm{ax}, \quad \mathrm{a} \neq 0$
$\Rightarrow \mathrm{ax}=0 \quad$ or $\mathrm{x}=0$
$\therefore 0$ is zero of $\mathrm{p}(\mathrm{x})$
(vii) $\mathrm{p}(\mathrm{x})=\mathrm{cx}+\mathrm{d}, \mathrm{c} \neq 0, \mathrm{c}, \mathrm{d}$ are real numbers
$c x+d=0 \Rightarrow c x=-d$
$\mathbf{x}=-\frac{\mathrm{d}}{\mathrm{c}}$’
$\therefore-\frac{d}{c}$ is zero of polynomial $p(x)$.

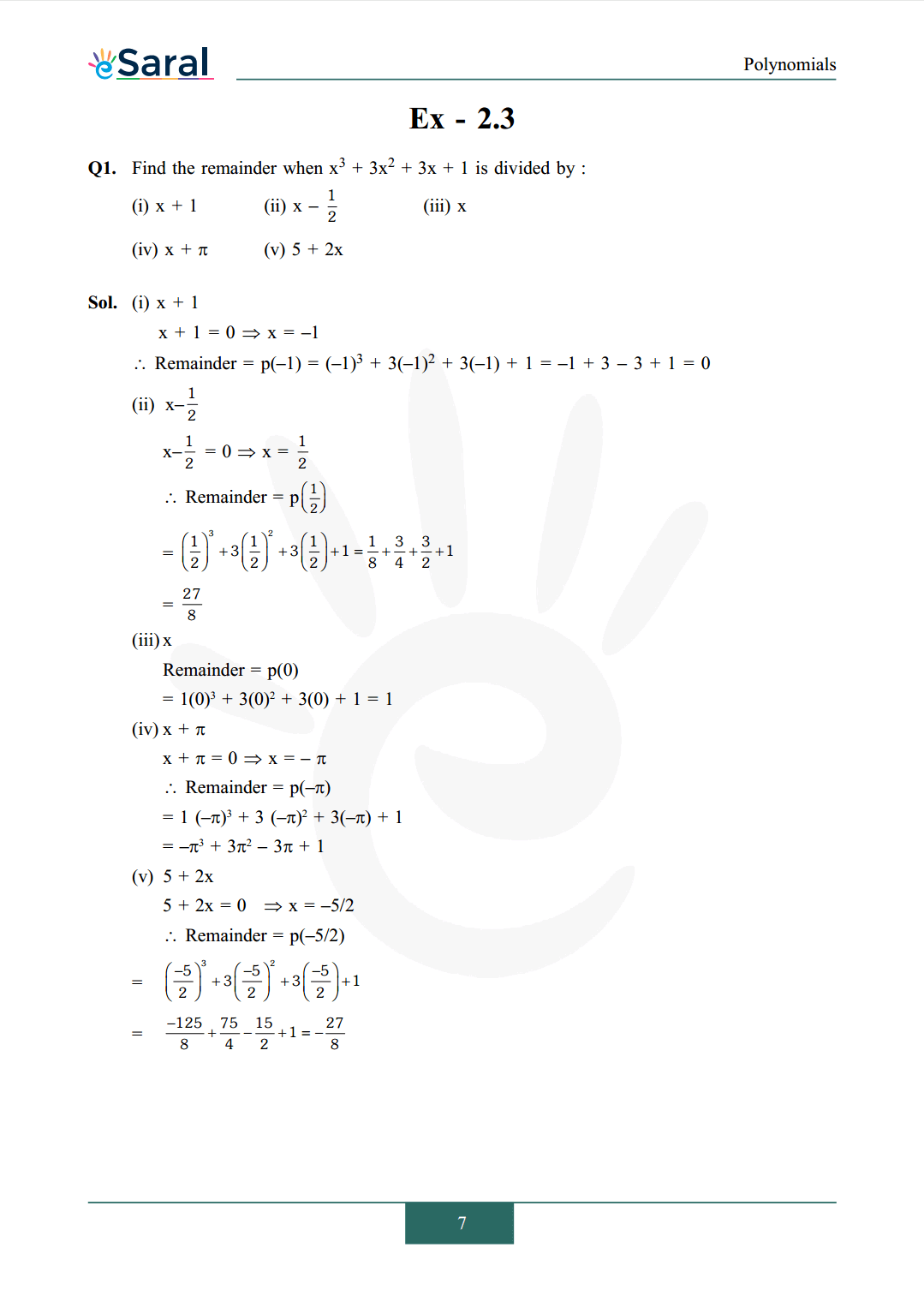
NCERT Solutions for Class 9 Maths chapter 2 Ex - 2.3

Q1. Find the remainder when $\mathrm{x}^{3}+3 \mathrm{x}^{2}+3 \mathrm{x}+1$ is divided by :
(i) $x+1$
(ii) $\mathrm{x}-\frac{1}{2}$
(iii) $\mathrm{X}$
(iv) $x+\pi$
(v) $5+2 \mathrm{x}$

Sol. (i) $x+1$
$x+1=0 \Rightarrow x=-1$
$\therefore$ Remainder $=\mathrm{p}(-1)=(-1)^{3}+3(-1)^{2}+3(-1)+1$
$=-1+3-3+1=0$
(ii) $\mathrm{x}-\frac{1}{2}$
$\mathrm{x}-\frac{1}{2}=0 \Rightarrow \mathrm{x}=\frac{1}{2}$
$\therefore$ Remainder $=\mathrm{p}\left(\frac{1}{2}\right)$
$=\left(\frac{1}{2}\right)^{3}+3\left(\frac{1}{2}\right)^{2}+3\left(\frac{1}{2}\right)$
$+1=\frac{1}{8}+\frac{3}{4}+\frac{3}{2}+1$
$=\frac{27}{8}$
(iii) $\mathrm{X}$
Remainder $=\mathrm{p}(0)$
$=1(0)^{3}+3(0)^{2}+3(0)+1=1$
(iv) $x+\pi$
$\mathrm{x}+\pi=0 \Rightarrow \mathrm{x}=-\pi$
$\therefore$ Remainder $=\mathrm{p}(-\pi)$
$=1(-\pi)^{3}+3(-\pi)^{2}+3(-\pi)+1$
$=-\pi^{3}+3 \pi^{2}-3 \pi+1$
(v) $5+2 \mathrm{x}$
$5+2 \mathrm{x}=0 \Rightarrow \mathrm{x}=-5 / 2$
$\therefore$ Remainder $=\mathrm{p}(-5 / 2)$
$=\left(\frac{-5}{2}\right)^{3}+3\left(\frac{-5}{2}\right)^{2}+3\left(\frac{-5}{2}\right)+1$
$=\frac{-125}{8}+\frac{75}{4}-\frac{15}{2}+1=-\frac{27}{8}$

Q2. Find the remainder when $x^{3}-a x^{2}+6 x-$ a divided by $x-a$.

Sol. Let $p(x)=x^{3}-a x^{2}+6 x-a$
$\mathrm{x}-\mathrm{a}=0 \Rightarrow \mathrm{x}=\mathrm{a}$
$\therefore$ Remainder $=(\mathrm{a})^{3}-\mathrm{a}(\mathrm{a})^{2}+6(\mathrm{a})-\mathrm{a}$
$=a^{3}-a^{3}+6 a-a=5 a$

Q3. Check whether $7+3 \mathrm{x}$ is a factor of $3 \mathrm{x}^{3}+7 \mathrm{x}$

Sol. $7+3 x$ will be a factor of $3 x^{3}+7 x$ only if
$7+3 x$ divides $3 x^{3}+7 x$ leaving 0 as remainder.
Let $p(x)=3 x^{3}+7 x$
$7+3 x=0 \Rightarrow 3 x=-7 \Rightarrow x=-7 / 3$
$\therefore$ Remainder
$3\left(-\frac{7}{3}\right)^{3}+7\left(-\frac{7}{3}\right)=\frac{-343}{9}-\frac{49}{3}=\frac{-490}{9} \neq 0$
so, $7+3 \mathrm{x}$ is not a factor of $3 \mathrm{x}^{3}+7 \mathrm{x}$.

NCERT Solutions for Class 9 Maths chapter 2 Ex - 2.4

Q1. Determine which of the following polynomials, $(x+1)$ is a factor of :
(i) $x^{3}+x^{2}+x+1$
(ii) $x^{4}+x^{3}+x^{2}+x+1$
(iii) $x^{4}+3 x^{3}+3 x^{2}+x+1$
(iv) $x^{3}-x^{2}-(2+\sqrt{2}) x+\sqrt{2}$

Sol.(i) $x^{3}+x^{2}+x+1$
Let $p(x)=x^{3}+x^{2}+x+1$
The zero of $x+1$ is $-1$
$\mathrm{p}(-1)=(-1)^{3}+(-1)^{2}+(-1)+1$
$=-1+1-1+1=0$
By Factor theorem $x+1$ is a factor of $p(x)$.
(ii) $x^{4}+x^{3}+x^{2}+x+1$
Let $\mathrm{p}(\mathrm{x})=\mathrm{x}^{4}+\mathrm{x}^{3}+\mathrm{x}^{2}+\mathrm{x}+1$
The zero of $x+1$ is $-1$
$p(-1)=(-1)^{4}+(-1)^{3}+(-1)^{2}+(-1)+1=1 \neq 0$
By Factor theorem $x+1$ is not a factor of $p(x)$
(iii) $x^{4}+3 x^{3}+3 x^{2}+x+1$
Let $p(x)=x^{4}+3 x^{3}+3 x^{2}+x+1$
Zero of $x+1$ is $-1$
$p(-1)=(-1)^{4}+3(-1)^{3}+3(-1)^{2}+(-1)+1$
$=1-3+3-1+1=1 \neq 0$
By Factor theorem $\mathrm{x}+1$ is not a factor of $\mathrm{p}(\mathrm{x})$
(iv) Let $\mathrm{p}(\mathrm{x})=\mathrm{x}^{3}-\mathrm{x}^{2}-(2+\sqrt{2}) \mathrm{x}+\sqrt{2}$
zero of $x+1$ is $-1$
$\mathrm{p}(-1)=(-1)^{3}-(-1)^{2}-(2+\sqrt{2})(-1)+\sqrt{2}$
$=-1-1+2+\sqrt{2}+\sqrt{2}=2 \sqrt{2} \neq 0$
By Factor theorem, $x+1$ is not a factor of $p(x)$.

Q2. Use the factor theorem to determine whether $\mathrm{g}(\mathrm{x})$ is a factor of $\mathrm{p}(\mathrm{x})$ in each of the following cases:
(i) $p(x)=2 x^{3}+x^{2}-2 x-1, g(x)=x+1$.
(ii) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{3}+3 \mathrm{x}^{2}+3 \mathrm{x}+1, \mathrm{~g}(\mathrm{x})=\mathrm{x}+2$.
(iii) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{3}-4 \mathrm{x}^{2}+\mathrm{x}+6 ; \mathrm{g}(\mathrm{x})=\mathrm{x}-3$

Sol. (i) $\mathrm{p}(\mathrm{x})=2 \mathrm{x}^{3}+\mathrm{x}^{2}-2 \mathrm{x}-1, \mathrm{~g}(\mathrm{x})=\mathrm{x}+1$.
$\mathrm{g}(\mathrm{x})=0 \Rightarrow \mathrm{x}+1=0 \Rightarrow \mathrm{x}=-1$
$\therefore$ Zero of $g(x)$ is $-1$
Now, $p(-1)=2(-1)^{3}+(-1)^{2}-2(-1)-1$
$=-2+1+2-1=0$
$\therefore$ By factor theorem, $g(x)$ is a factor of $p(x)$.
(ii) Let $\mathrm{p}(\mathrm{x})=\mathrm{x}^{3}+3 \mathrm{x}^{2}+3 \mathrm{x}+1$,
$g(x)=x+2$
$g(x)=0 \Rightarrow x+2=0$
$\Rightarrow \mathrm{x}=-2$
$\therefore$ Zero of $g(x)$ is $-2$
Now, $\mathrm{p}(-2)=(-2)^{3}+3(-2)^{2}+3(-2)+1$
$=-8+12-6+1=-1$
$\therefore$ By Factor theorem, $\mathrm{g}(\mathrm{x})$ is not a factor of $\mathrm{p}(\mathrm{x})$
(iii) $p(x)=x^{3}-4 x^{2}+x+6, g(x)=x-3$
$g(x)=0$
$\Rightarrow x-3=0 \Rightarrow x=3$
$\therefore$ Zero of $g(x)=3$
Now $\mathrm{p}(3)=3^{3}-4(3)^{2}+3+6$
$=27-36+3+6=0$
$\therefore$ By Factor theorem, $\mathrm{g}(\mathrm{x})$ is a factor of $\mathrm{p}(\mathrm{x})$.

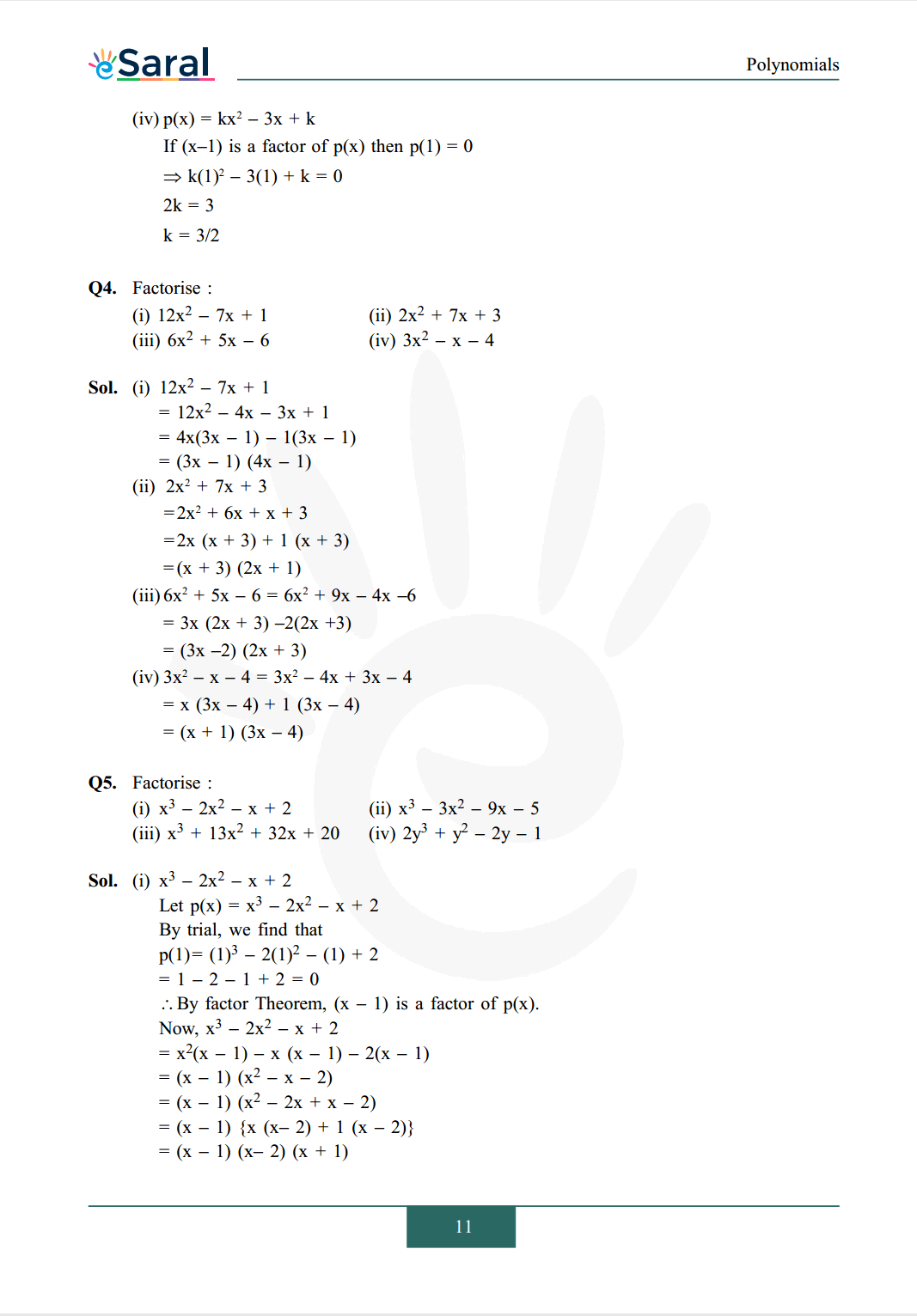
Q3. Find the value of $\mathrm{k}$, if $\mathrm{x}-1$ is a factor of $\mathrm{p}(\mathrm{x})$ in each of the following cases :
(i) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{2}+\mathrm{x}+\mathrm{k}$
(iii) $\mathrm{p}(\mathrm{x})=\mathrm{kx}^{2}-\sqrt{2} \mathrm{x}+1$
(iv) $p(x)=k x^{2}-3 x+k$

Sol. (i) $\mathrm{p}(\mathrm{x})=\mathrm{x}^{2}+\mathrm{x}+\mathrm{k}$
If $x-1$ is a factor of $p(x)$, then $p(1)=0$
$\Rightarrow(1)^{2}+(1)+\mathrm{k}=0$
$\Rightarrow 1+1+K=0$ $\Rightarrow 2+\mathrm{k}=0$
$\Rightarrow \mathrm{k}=-2$
(ii) $\mathrm{p}(\mathrm{x})=2 \mathrm{x}^{2}+\mathrm{kx}+\sqrt{2}$
If $(x-1)$ is a factor of $p(x)$ then $p(1)=0$
$\Rightarrow 2(1)^{2}+\mathrm{k}(1)+\sqrt{2}=0$
$\Rightarrow 2+\mathrm{k}+\sqrt{2}=0$
$\mathrm{k}=-(2+\sqrt{2})$
(iii) $p(x)=k x^{2}-\sqrt{2} x+1$
If $(x-1)$ is a factor of $p(x)$ then $p(1)=0$
$\mathrm{k}(1)^{2}-\sqrt{2}(1)+1=0$
$\Rightarrow \mathrm{k}-\sqrt{2}+1=0$
$\mathrm{k}=\sqrt{2}-1$
(iv) $\mathrm{p}(\mathrm{x})=\mathrm{kx}^{2}-3 \mathrm{x}+\mathrm{k}$
If $(x-1)$ is a factor of $p(x)$ then $p(1)=0$
$\Rightarrow \mathrm{k}(1)^{2}-3(1)+\mathrm{k}=0$
$2 \mathrm{k}=3$
$\mathrm{k}=3 / 2$

Q4. Factorise :
(i) $12 \mathrm{x}^{2}-7 \mathrm{x}+1$
(ii) $2 \mathrm{x}^{2}+7 \mathrm{x}+3$
(iii) $6 x^{2}+5 x-6$
(iv) $3 x^{2}-x-4$

Sol. (i) $12 \mathrm{x}^{2}-7 \mathrm{x}+1$
$=12 x^{2}-4 x-3 x+1$
$=4 x(3 x-1)-1(3 x-1)$
$=(3 x-1)(4 x-1)$
(ii) $2 x^{2}+7 x+3$
$=2 x^{2}+6 x+x+3$
$=2 \mathrm{x}(\mathrm{x}+3)+1(\mathrm{x}+3)$
$=(\mathrm{x}+3)(2 \mathrm{x}+1)$
(iii) $6 x^{2}+5 x-6=6 x^{2}+9 x-4 x-6$
$=3 x(2 x+3)-2(2 x+3)$
$=(3 x-2)(2 x+3)$
(iv) $3 x^{2}-x-4=3 x^{2}-4 x+3 x-4$
$=x(3 x-4)+1(3 x-4)$
$=(x+1)(3 x-4)$

Q5. Factorise :
(i) $x^{3}-2 x^{2}-x+2$
(ii) $x^{3}-3 x^{2}-9 x-5$
(iii) $x^{3}+13 x^{2}+32 x+20$
(iv) $2 \mathrm{y}^{3}+\mathrm{y}^{2}-2 \mathrm{y}-1$

Sol. (i) $x^{3}-2 x^{2}-x+2$
Let $p(x)=x^{3}-2 x^{2}-x+2$
By trial, we find that
$\mathrm{p}(1)=(1)^{3}-2(1)^{2}-(1)+2$
$=1-2-1+2=0$
$\therefore$ By factor Theorem, $(\mathrm{x}-1)$ is a factor of $\mathrm{p}(\mathrm{x})$.
Now, $x^{3}-2 x^{2}-x+2$
$=x^{2}(x-1)-x(x-1)-2(x-1)$
$=(x-1)\left(x^{2}-x-2\right)$
$=(x-1)\left(x^{2}-2 x+x-2\right)$
$=(x-1)\{x(x-2)+1(x-2)\}$
$=(x-1)(x-2)(x+1)$
(ii) $x^{3}-3 x^{2}-9 x-5$

Let $p(x)=x^{3}-3 x^{2}-9 x-5$
Bv trial. we find
$\mathrm{p}(-1)=(-1)^{3}-3(-1)^{2}-9(-1)-5$
$=-1-3+9-5=0$
$\therefore$ By Factor Theorem, $x-(-1)$ or $x+1$ is factor of $p(x)$
Now, $x^{3}-3 x^{2}-9 x-5$

$=x^{2}(x+1)-4 x(x+1)-5(x+1)$
$=(x+1)\left(x^{2}-4 x-5\right)$
$=(x+1)\left(x^{2}-5 x+x-5\right)$
$=(x+1)\{x(x-5)+1(x-5)\}$
$=(x+1)^{2}(x-5)$
(iii) $x^{3}+13 x^{2}+32 x+20$
Let $p(x)=x^{3}+13 x^{2}+32 x+20$
By trial, we find
$p(-1)=(-1)^{3}+13(-1)^{2}+32(-1)+20$
$=-1+13-32+20=0$
$\therefore$ By Factor theorem, $x-(-1), x+1$ is a factor of $p(x)$
$x^{3}+13 x^{2}+32 x+20$
$=x^{2}(x+1)+12(x)(x+1)+20(x+1)$
$=(x+1)\left(x^{2}+12 x+20\right)$
$=(x+1)\left(x^{2}+2 x+10 x+20\right)$
$=(x+1)\{x(x+2)+10(x+2)\}$
$=(x+1)(x+2)(x+10)$
(iv) $2 \mathrm{y}^{3}+\mathrm{y}^{2}-2 \mathrm{y}-1$
$p(y)=2 y^{3}+y^{2}-2 y-1$’
By trial, we find that
$p(1)=2(1)^{3}+(1)^{2}-2(1)-1=0$
$\therefore$ By Factor theorem, $(\mathrm{y}-1)$ is a factor of $\mathrm{p}(\mathrm{y})$
$2 y^{3}+y^{2}-2 y-1$
$=2 y^{2}(y-1)+3 y(y-1)+1(y-1)$
$=(y-1)\left(2 y^{2}+3 y+1\right)$
$=(y-1)\left(2 y^{2}+2 y+y+1\right)$
$=(\mathrm{y}-1)\{2 \mathrm{y}(\mathrm{y}+1)+1(\mathrm{y}+1)\}$
$=(\mathrm{y}-1)(2 \mathrm{y}+1)(\mathrm{y}+1)$

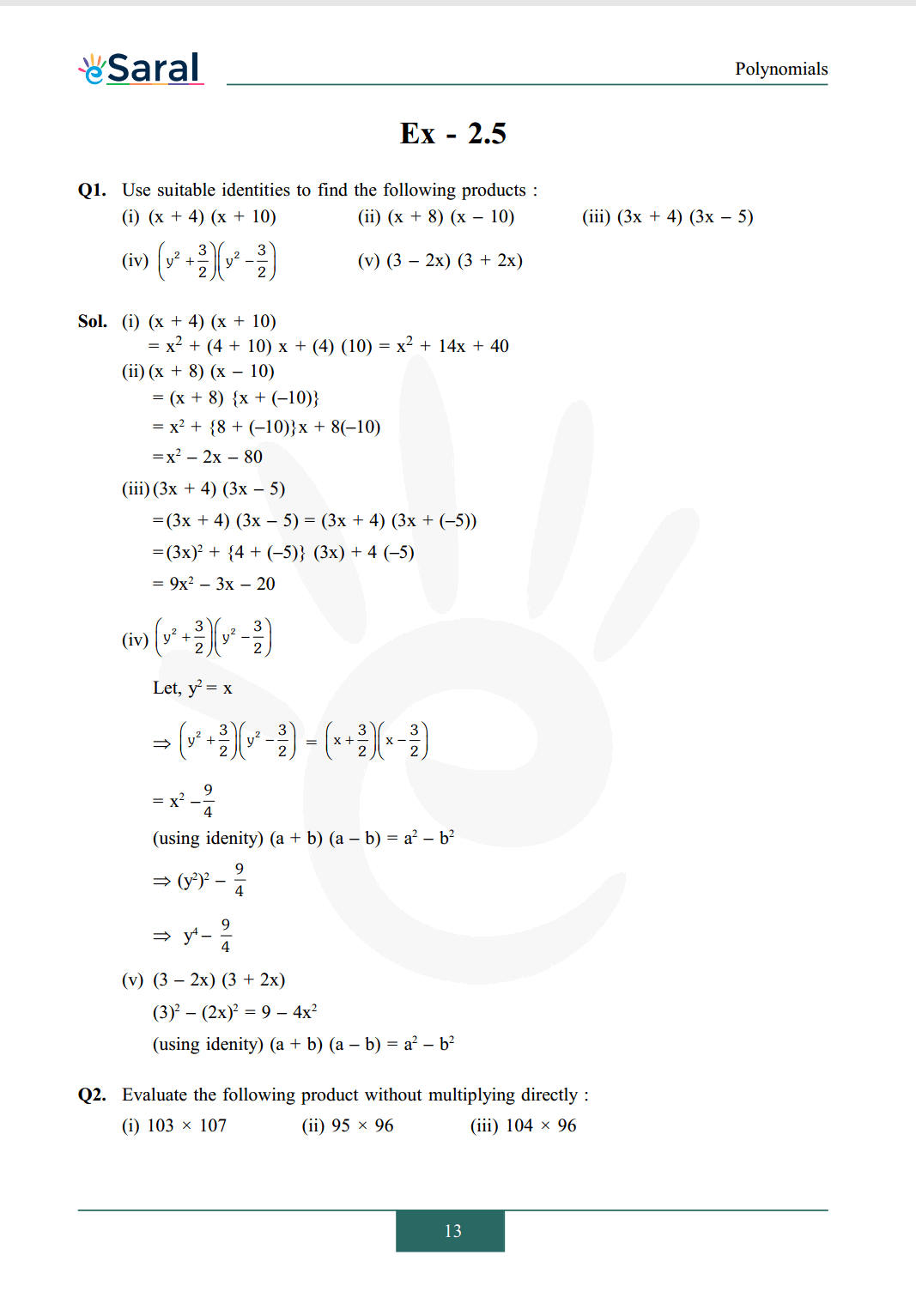
NCERT Solutions for Class 9 Maths chapter 2 Ex - 2.5

Q1. Use suitable identities to find the following products:
(i) $(x+4)(x+10)$
(ii) $(x+8)(x-10)$
(iii) $(3 x+4)(3 x-5)$
(iv) $\left(y^{2}+\frac{3}{2}\right)\left(y^{2}-\frac{3}{2}\right)$
(v) $(3-2 x)(3+2 x)$

Sol. (i) $(\mathrm{x}+4)(\mathrm{x}+10)$
$=x^{2}+(4+10) x+(4)(10)=x^{2}+14 x+40$
(ii) $(x+8)(x-10)$
$=(\mathrm{x}+8)\{\mathrm{x}+(-10)\}$
$=x^{2}+\{8+(-10)\} x+8(-10)$
$=x^{2}-2 x-80$
(iii) $(3 x+4)(3 x-5)$
$=(3 x+4)(3 x-5)=(3 x+4)(3 x+(-5))$
$=(3 x)^{2}+\{4+(-5)\}(3 x)+4(-5)$
$=9 x^{2}-3 x-20$
(iv) $\left(y^{2}+\frac{3}{2}\right)\left(y^{2}-\frac{3}{2}\right)$
Let, $y^{2}=x$
$\Rightarrow\left(y^{2}+\frac{3}{2}\right)\left(y^{2}-\frac{3}{2}\right)=\left(x+\frac{3}{2}\right)\left(x-\frac{3}{2}\right)$
$=x^{2}-\frac{9}{4}$
(using idenity) $(a+b)(a-b)=a^{2}-b^{2}$
$\Rightarrow\left(\mathrm{y}^{2}\right)^{2}-\frac{9}{4}$
$\Rightarrow \mathrm{y}^{4}-\frac{9}{4}$
(v) $(3-2 \mathrm{x})(3+2 \mathrm{x})$
$(3)^{2}-(2 x)^{2}=9-4 x^{2}$
(using idenity) $(a+b)(a-b)=a^{2}-b^{2}$

Q2. Evaluate the following product without multiplying directly:
(i) $103 \times 107$
(ii) $95 \times 96$
(iii) $104 \times 96$

Sol. (i) $103 \times 107=(100+3) \times(100+7)$
$=(100)^{2}+(3+7)(100)+(3)(7)$
$=10000+1000+21=11021$
Alternate solution :
$103 \times 107=(105-2) \times(105+2)$
$=(105)^{2}-(2)^{2}=(100+5)^{2}-4$
$=(100)^{2}+2(100)(5)+(5)^{2}-4$
$=10000+1000+25-4$
$=11021 .$
(ii) $95 \times 96$
$=(90+5) \times(90+6)$
$=(90)^{2}+(5+6) 90+(5)(6)$
$=8100+990+30=9120$
(iii) $104 \times 96$
$=(100+4) \times(100-4)$
(using idenity) $(a+b)(a-b)=a^{2}-b^{2}$
$=(100)^{2}-(4)^{2}=10000-16$
$=9984$

Q3. Factorise the following using appropriate identities:
(i) $9 x^{2}+6 x y+y^{2}$
(ii) $4 y^{2}-4 y+1$
(iii) $\mathrm{x}^{2}-\frac{\mathrm{y}^{2}}{100}$

Sol. (i) $9 x^{2}+6 x y+y^{2}=(3 x)^{2}+2(3 x)(y)+(y)^{2}$
$=(3 \mathrm{x}+\mathrm{y})^{2}$
$=(3 x+y)(3 x+y)$
(ii) $4 \mathrm{y}^{2}-4 \mathrm{y}+1$
$=(2 y)^{2}-2(2 y)(1)+(1)^{2}$
$=(2 y-1)^{2}=(2 y-1)(2 y-1)$
(iii) $\mathrm{x}^{2}-\frac{\mathrm{y}^{2}}{100}$
(using idenity) $a^{2}-b^{2}=(a+b)(a-b)$
$x^{2}-\left(\frac{y}{10}\right)^{2}=\left(x+\frac{y}{10}\right)\left(x-\frac{y}{10}\right)$

Q4. Expand each of the following using suitable identities:
(i) $(\mathrm{x}+2 \mathrm{y}+4 \mathrm{z})^{2}$
(ii) $(2 \mathrm{x}-\mathrm{y}+\mathrm{z})^{2}$
(iii) $(-2 x+3 y+2 z)^{2}$
(iv) $(3 a-7 b-c)^{2}$
(v) $(-2 x+5 y-3 z)^{2}$
(vi) $\left[\frac{1}{4} \mathrm{a}-\frac{1}{2} \mathrm{~b}+1\right]^{2}$

Sol. (i) $(\mathrm{x}+2 \mathrm{y}+4 \mathrm{z})^{2}=(\mathrm{x})^{2}+(2 \mathrm{y})^{2}$
$+(4 z)^{2}+2(x)(2 y)$
$2(2 y)(4 z)+2(4 z)(x)$
$=x^{2}+4 y^{2}+16 z^{2}+4 x y+16 y z+8 z x$
(ii) $(2 x-y+z)^{2}$
$=(2 x-y+z)(2 x-y+z)$
$=(2 x)^{2}+(-y)^{2}+(z)^{2}+2(2 x)(-y)$
$+2(-y)(z)+2(z)(2 x)$
$=4 \mathrm{x}^{2}+\mathrm{y}^{2}+\mathrm{z}^{2}-4 \mathrm{xy}-2 \mathrm{yz}+4 \mathrm{zx}$
(iii) $(-2 x+3 y+2 z)^{2}$
$=(-2 x)^{2}+(3 y)^{2}+(2 z)^{2}+2(-2 x)(3 y)$
$+2(-2 x)(2 z)+2(3 y)(2 z)$
$=4 x^{2}+9 y^{2}+4 z^{2}-12 x y-8 x z+12 y z$
(iv) $(3 a-7 b-c)^{2}=(3 a-7 b-c)(3 a-7 b-c)$
$=(3 \mathrm{a})^{2}+(-7 \mathrm{~b})^{2}+(-\mathrm{c})^{2}+2(3 \mathrm{a})(-7 \mathrm{~b})+$
$2(3 a)(-c)+2(-7 b)(-c)$
$=9 a^{2}+49 b^{2}+c^{2}-42 a b-6 a c+14 b c$
(v) $(-2 x+5 y-3 z)^{2}$
$=(-2 x+5 y-3 z)(-2 x+5 y-3 z)$
$=(-2 x)^{2}+(5 y)^{2}+(-3 z)^{2}+2(-2 x)(5 y)+$
$2(-2 x)(-3 z)+2(-3 z)(5 y)$
$=4 x^{2}+25 y^{2}+9 z^{2}-20 x y+12 x z-30 y z$
(vi) $\left[\frac{1}{4} \mathrm{a}-\frac{1}{2} \mathrm{~b}+1\right]^{2}$
$=\left(\frac{1}{4} a-\frac{1}{2} b+1\right)\left(\frac{1}{4} a-\frac{1}{2} b+1\right)$
$=\left(\frac{1}{4} a\right)^{2}+\left(-\frac{1}{2} b\right)^{2}+(1)^{2}+2\left(\frac{1}{4} a\right)$
$\left(-\frac{1}{2} b\right)+2\left(\frac{1}{4} a\right)(1)^{2}+2\left(-\frac{1}{2} b\right)(1)$
$=\frac{1}{16} a^{2}+\frac{1}{4} b^{2}+1-\frac{1}{4} a b-b+\frac{1}{2} a$

Q5. Factorise:
(i) $4 x^{2}+9 y^{2}+16 z^{2}+12 x y-24 y z-16 x z$
(ii) $2 \mathrm{x}^{2}+\mathrm{y}^{2}+8 \mathrm{z}^{2}-2 \sqrt{2} \mathrm{xy}+4 \sqrt{2} \mathrm{yz}-8 \mathrm{zx}$

Sol. (i) $4 x^{2}+9 y^{2}+16 z^{2}+12 x y-24 y z-16 x z$
$=(2 x)^{2}+(3 y)^{2}+(-4 z)^{2}+2(2 x)(3 y)+2(3 y)(-4 z)+2(-4 z)(-2 x)$
$=\{2 \mathrm{x}+3 \mathrm{y}+(-4 \mathrm{z})\}^{2}=(2 \mathrm{x}+3 \mathrm{y}-4 \mathrm{z})^{2}$
$=(2 x+3 y-4 z)(2 x+3 y-4 z)$
(ii) $2 \mathrm{x}^{2}+\mathrm{y}^{2}+8 \mathrm{z}^{2}-2 \sqrt{2} \mathrm{xy}+4 \sqrt{2} \mathrm{yz}-8 \mathrm{zx}$
$=(-\sqrt{2} \mathrm{x})^{2}+\mathrm{y}^{2}+(2 \sqrt{2} \mathrm{z})^{2}+2(-\sqrt{2} \mathrm{x})$
$y+2 y(2 \sqrt{2} z)+2(2 \sqrt{2} z)(-\sqrt{2} x)$
$=(-\sqrt{2} x+y+2 \sqrt{2} z)^{2}$

Q6. Write the following cubes in expanded form :
(i) $(2 x+1)^{3}$
(ii) $(2 \mathrm{a}-3 \mathrm{~b})^{3}$
(iii) $\left[\frac{3}{2} \mathrm{x}+1\right]^{3}$
(iv) $\left[x-\frac{2}{3} y\right]^{3}$

Sol. (i) $(2 x+1)^{3}=(2 x)^{3}+(1)^{3}+3(2 x)(1)(2 x+1)$
$=8 x^{3}+1+6 x(2 x+1)$
$=8 x^{3}+1+12 x^{2}+6 x$
$=8 x^{3}+12 x^{2}+6 x+1$
(ii) $(2 a-3 b)^{3}=(2 a)^{3}-(3 b)^{3}-3(2 a)(3 b)(2 a-3 b)$
$=8 a^{3}-27 b^{3}-18 a b(2 a-3 b)$
$=8 a^{3}-27 b^{3}-36 a^{2} b+54 a b^{2}$
(iii) $\left[\frac{3}{2} x+1\right]^{3}=\left(\frac{3}{2} x\right)^{3}+(1)^{3}$
$3\left(\frac{3}{2} x\right)(1)\left(\frac{3}{2} x+1\right)$
$=\frac{27}{8} \mathrm{x}^{3}+1+\frac{27}{4} \mathrm{x}^{2}+\frac{9}{2} \mathrm{x}$
$=\frac{27}{8} \mathrm{x}^{3}+\frac{27}{4} \mathrm{x}^{2}+\frac{9}{2} \mathrm{x}+1$
(iv) $\left(x-\frac{2}{3} y\right)^{3}=x^{3}-\left(\frac{2}{3} y\right)^{3}$
$-3 x\left(\frac{2}{3} y\right)\left(x-\frac{2}{3} y\right)$
$=x^{3}-\frac{8}{27} y^{3}-2 x y\left(x-\frac{2}{3} y\right)$
$=x^{3}-\frac{8}{27} y^{3}-2 x^{2} y+\frac{4}{3} x y^{2}$

Q7. Evaluate the following using suitable identities:
(i) $(99)^{3}$
(ii) $(102)^{3}$
(iii) $(998)^{3}$

Sol.(i) $(99)^{3}=(100-1)^{3}$
$=(100)^{3}-(1)^{3}-3(100)(1)(100-1)$
$=1000000-1-300(100-1)$
$=1000000-1-30000+300$
$=970299$
(ii) $(102)^{3}=(100+2)^{3}$
$=(100)^{3}+(2)^{3}+3(100)(2)(100+2)$
$=1000000+8+600(100+2)$
v$=1000000+8+60000+1200$
$=1061208$
(iii) $(998)^{3}=(1000-2)^{3}$
$=(1000)^{3}-(2)^{3}-3(1000)(2)(1000-2)$
$=1000000000-8-6000(1000-2)$
$=994011992$

Q8. Factorise each of the following :
(i) $8 a^{3}+b^{3}+12 a^{2} b+6 a b^{2}$
(ii) $8 \mathrm{a}^{3}-\mathrm{b}^{3}-12 \mathrm{a}^{2} \mathrm{~b}+6 \mathrm{ab}^{2}$
(iii) $27-125 \mathrm{a}^{3}-135 \mathrm{a}+225 \mathrm{a}^{2}$
(iv) $64 \mathrm{a}^{3}-27 \mathrm{~b}^{3}-144 \mathrm{a}^{2} \mathrm{~b}+108 \mathrm{ab}^{2}$
(v) $27 \mathrm{p}^{3}-\frac{1}{216}-\frac{9}{2} \mathrm{p}^{2}+\frac{1}{4} \mathrm{p}$

Sol.(i) $8 \mathrm{a}^{3}+\mathrm{b}^{3}+12 \mathrm{a}^{2} \mathrm{~b}+6 \mathrm{ab}^{2}$
$=(2 a)^{3}+(b)^{3}+3(2 a)(b)(2 a+b)$
$=(2 a+b)^{3}=(2 a+b)(2 a+b)(2 a+b)$
(ii) $8 \mathrm{a}^{3}-\mathrm{b}^{3}-12 \mathrm{a}^{2} \mathrm{~b}+6 \mathrm{ab}^{2}$
$=(2 a)^{3}+(-b)^{3}+3(2 a)^{2}(-b)+3(2 a)(-b)^{2}$
$=(2 a-b)^{3}$
(iii) $27-125 \mathrm{a}^{3}-135 \mathrm{a}+225 \mathrm{a}^{2}$
$=3^{3}-(5 a)^{3}-3(3)(5 a)(3-5 a)$
$=(3-5 a)^{3}$
(iv) $64 \mathrm{a}^{3}-27 \mathrm{~b}^{3}-144 \mathrm{a}^{2} \mathrm{~b}+180 \mathrm{ab}^{2}$
$=(4 a)^{3}-(3 b)^{3}-3(4 a)(3 b)(4 a-3 b)$
$=(4 a-3 b)^{3}$
(v) $27 \mathrm{p}^{3}-\frac{1}{216}-\frac{9}{2} \mathrm{p}^{2}+\frac{1}{4 \mathrm{p}}$
$=(3 p)^{3}-\left(\frac{1}{6}\right)^{3}-3(3 p)\left(\frac{1}{6}\right)\left(3 p-\frac{1}{6}\right)$
$=\left(3 p-\frac{1}{6}\right)^{3}=\left(3 p-\frac{1}{6}\right)\left(3 p-\frac{1}{6}\right)\left(3 p-\frac{1}{6}\right)$

Q9. Verify : (i) $x^{3}+y^{3}=(x+y)\left(x^{2}-x y+y^{2}\right)$
(ii) $\mathrm{x}^{3}-\mathrm{y}^{3}=(\mathrm{x}-\mathrm{y})\left(\mathrm{x}^{2}+\mathrm{xy}+\mathrm{y}^{2}\right)$

Sol. (i) $(x+y)^{3}=x^{3}+y^{3}+3 x y(x+y)$
$\Rightarrow x^{3}+y^{3}=(x+y)^{3}-3 x y(x+y)$
$\Rightarrow \mathrm{x}^{3}+\mathrm{y}^{3}=(\mathrm{x}+\mathrm{y})\left\{(\mathrm{x}+\mathrm{y})^{2}-3 \mathrm{xy}\right\}$
$\Rightarrow x^{3}+y^{3}=(x+y)\left(x^{2}+2 x y+y^{2}-3 x y\right)$
$\Rightarrow x^{3}+y^{3}=(x+y)\left(x^{2}-x y+y^{2}\right)$
(ii) $(x-y)^{3}=x^{3}-y^{3}-3 x y(x-y)$
$\Rightarrow x^{3}-y^{3}=(x-y)^{3}+3 x y(x-y)$
$\Rightarrow x^{3}-y^{3}=(x-y)\left[(x-y)^{2}+3 x y\right]$
$\Rightarrow x^{3}-y^{3}=(x-y)\left[x^{2}+y^{2}-2 x y+3 x y\right]$
$\Rightarrow x^{3}-y^{3}=(x-y)\left[x^{2}+y^{2}+x y\right]$

Q10. Factorise each of the following :
(i) $27 \mathrm{y}^{3}+125 \mathrm{z}^{3}$
(ii) $64 \mathrm{~m}^{3}-343 \mathrm{n}^{3}$

Sol.(i) $27 \mathrm{y}^{3}+125 \mathrm{z}^{3}=(3 \mathrm{y})^{3}+(5 \mathrm{z})^{3}$
$=(3 y+5 z)\left\{(3 y)^{2}-(3 y)(5 z)+(5 z)^{2}\right\}$
$=(3 y+5 z)\left(9 y^{2}-15 y z+25 z^{2}\right)$
(ii) $64 \mathrm{~m}^{3}-343 \mathrm{n}^{3}$
$=(4 m)^{3}-(7 n)^{3}$
$=[4 m-7 n]\left[16 m^{2}+4 m .7 n+(7 n)^{2}\right]$
$=(4 m-7 n)\left[16 m^{2}+28 m n+49 n^{2}\right]$

Q11. Factorise : $27 \mathrm{x}^{3}+\mathrm{y}^{3}+\mathrm{z}^{3}-9 \mathrm{xyz}$

Sol. $27 \mathrm{x}^{3}+\mathrm{y}^{3}+\mathrm{z}^{3}-9 \mathrm{xyz}$
$=(3 x)^{3}+(y)^{3}+(z)^{3}-3(3 x)(y)(z)$
$=(3 x+y+z)\left((3 x)^{2}+(y)^{2}+(z)^{2}\right.$
$-(3 x)(y)-(y)(z)-(z)(3 x))$
$=(3 x+y+z)\left(9 x^{2}+y^{2}+z^{2}-3 x y-y z-3 z x\right)$

Q12. Verify that $\mathrm{x}^{3}+\mathrm{y}^{3}+\mathrm{z}^{3}-3 \mathrm{xyz}$
$=\frac{1}{2}(x+y+z)\left[(x-y)^{2}+(y-z)^{2}+(z-x)^{2}\right]$

Sol. $\frac{1}{2}(x+y+z)\left[(x-y)^{2}+(y-z)^{2}+(z-x)^{2}\right]$
$=\frac{1}{2}(x+y+z)\left[\left(x^{2}-2 x y+y^{2}\right)\right.$
$=-\frac{1}{2}(x+y+z)\left[\left(x^{2}-2 x y+y^{2}\right)\right.$
$+\left(y^{2}-2 y z+z^{2}\right)+\left(z^{2}-2 z x+x^{2}\right)$
$=\frac{1}{2}(x+y+z)\left[2 x^{2}+2 y^{2}+2 z^{2}-2 x y-2 y z-2 z x\right]$
$=\frac{1}{2}(x+y+z) 2\left(x^{2}+y^{2}+z^{2}-x y-y z-z x\right]$
$=(\mathrm{x}+\mathrm{y}+\mathrm{z})\left(\mathrm{x}^{2}+\mathrm{y}^{2}+\mathrm{z}^{2}-\mathrm{xy}-\mathrm{yz}-\mathrm{zx}\right]$
$=x^{3}+y^{3}+z^{3}-3 x y z$

Q13. If $x+y+z=0$, show that $x^{3}+y^{3}+z^{3}=3 x y z$

Sol. We know
$x^{3}+y^{3}+z^{3}-3 x y z$
$=(\mathrm{x}+\mathrm{y}+\mathrm{z})\left(\mathrm{x}^{2}+\mathrm{y}^{2}+\mathrm{z}^{2}-\mathrm{xy}-\mathrm{yz}-\mathrm{zx}\right)$
$x+y+z=0$ [given $]$
$\Rightarrow(0)\left(\mathrm{x}^{2}+\mathrm{y}^{2}+\mathrm{z}^{2}-\mathrm{xy}-\mathrm{yz}-\mathrm{zx}\right)$
$=0$
or $x^{3}+y^{3}+z^{3}=3 x y z$

Q14. Without actually calculating the cubes, find the value of each of the following :
(i) $(-12)^{3}+(7)^{3}+(5)^{3}$
(ii) $(28)^{3}+(-15)^{3}+(-13)^{3}$

Sol.(i) $(-12)^{3}+(7)^{3}+(5)^{3}$
$=\left\{(-12)^{3}+(7)^{3}+(5)^{3}-3(-12)(7)\right.$
$(5)\}+3(-12)(7)(5)$
$=(-12+7+5)\left\{(-12)^{2}+(7)^{2}+(5)^{2}-(-12)(7)\right.$
$-(7)(5)-(5)(-12)\}+3(-12)(7)(5)$
$=0+3(-12)(7)(5)=-1260$
(ii) $(28)^{3}+(-15)^{3}+(-13)^{3}$
$\because 28-15-13=0$
$(28)^{3}+(-15)^{3}+(-13)^{3}$
$=3(28)(-15)(-13)=16380$
(using identity)
if $a+b+c=0$
$\Rightarrow \mathrm{a}^{3}+\mathrm{b}^{3}+\mathrm{c}^{3}=3 \mathrm{abc}$

Q15. Give possible expressions for the length and breadth of each of the following rectangles, in which their areas are given :
(i) Area : $25 \mathrm{a}^{2}-35 \mathrm{a}+12$
(ii)Area : $35 \mathrm{y}^{2}+13 \mathrm{y}-12$

Sol. (i) Area $=25 a^{2}-35 a+12$
$=25 a^{2}-20 a-15 a+12$
$=5 \mathrm{a}(5 \mathrm{a}-4)-3(5 \mathrm{a}-4)$
$=(5 a-3)(5 a-4)$
Here, Length $=5 \mathrm{a}-3$, Breadth $=5 \mathrm{a}-4$

Q16. What are the possible expressions for the dimensions of the cuboids whose volumes are given below?
(i) Volume : $3 x^{2}-12 x$
(ii) Volume : $12 \mathrm{ky}^{2}+8 \mathrm{ky}-20 \mathrm{k}$

Sol. (i) Volume $=3 \mathrm{x}^{2}-12 \mathrm{x}$ss
$=3 x(x-4)=3 \times x(x-4)$
$\therefore$ Dimensions are 3 units, $x$ -units and $(x-4)$ units
(ii) $12 \mathrm{ky}^{2}+8 \mathrm{ky}-20 \mathrm{k}$
$=4 \mathrm{k}\left(3 \mathrm{y}^{2}+2 \mathrm{y}-5\right)=4 \mathrm{k}\left(3 \mathrm{y}^{2}+5 \mathrm{y}-3 \mathrm{y}-5\right)$
$=4 \mathrm{k}\{\mathrm{y}(3 \mathrm{y}+5)-1(3 \mathrm{y}+5)\}$
$=4 \mathrm{k}(3 \mathrm{y}+5)(\mathrm{y}-1)$
$\therefore$ Dimensions of cuboid are $4 \mathrm{k}, 3 \mathrm{y}+5, \mathrm{y}-1$

Chapter 2 - Polynomials

In this Chapter you will learn about the Variables and coefficients and there operations of Multiplication, subtraction, and Additions. After completing this chapter you will get the idea about Polynomials, coefficients, Degrees and different terms of Polynomials

NCERT Class 9 Maths Chapter 2 All Exercise Solutions

• Class 9 maths chapter 2 exercise 2.1
• Class 9 maths chapter 2 exercise 2.2
• Class 9 maths chapter 2 exercise 2.3
• Class 9 maths chapter 2 exercise 2.4
• Class 9 maths chapter 2 exercise 2.5

So, that’s all from this article. I hope you enjoyed this post. If you found this article helpful then please share it with other students.

Also Read,

Complete Class 9 Maths Short Revision Notes.

NCERT Class 9 Maths Book PDF

NCERT Class 9 Maths Exemplar

Also See.

• Class 9 Maths all chapter solutions.
• Chapter 1: Number System
• Chapter 2: Polynomials
• Chapter 3: Coordinate Geometry
• Chapter 4: Linear Equations in Two Variables
• Chapter 5: Introduction to Euclid’s Geometry
• Chapter 6: Lines and Angles
• Chapter 7: Triangles
• Chapter 8: Quadrilaterals
• Chapter 9: Areas of Parallelograms and Triangles
• Chapter 10: Circles
• Chapter 11: Constructions
• Chapter 12: Heron’s Formula
• Chapter 13: Surface Areas and Volumes
• Chapter 14: Statistics
• Chapter 15: Probability

If you have any Confusion related to NCERT Solutions for Class 9 Maths chapter 2 Polynomials PDF then feel free to ask in the comments section down below.

To watch Free Learning Videos on Class 9 by Kota’s top Faculties Install the eSaral App